Advertisements
Advertisements
प्रश्न
Factorise the following:
a4 – 3a2 + 2
उत्तर
Let a2 = x
a4 – 3a2 + 2 = (a2)2 – 3a2 + 2
= x2 – 3x + 2
Product = 2 and sum = – 3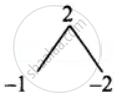
Split the middle term as – x and – 2x
x² – 3x + 2 = x2 – x – 2x + 2
= x(x – 1) – 2(x – 1)
= (x – 1)(x – 2)
a4 – 3a2 + 2 = (a2 – 1)(a2 – 2) ...[But a2 = x]
= (a + 1)(a – 1)(a2 – 2)
APPEARS IN
संबंधित प्रश्न
Identify constant, linear, quadratic and cubic polynomials from the following polynomials:
`h(x)=-3x+1/2`
Identify constant, linear, quadratic and cubic polynomials from the following polynomials:
`r(x)=3x^2+4x^2+5x-7`
Find rational roots of the polynomial f(x) = 2x3 + x2 − 7x − 6.
The polynomials ax3 + 3x2 − 3 and 2x3 − 5x + a when divided by (x − 4) leave the remainders R1 and R2 respectively. Find the value of the following case, if R1 = R2.
In the following two polynomials, find the value of a, if x − a is factor x6 − ax5 + x4 − ax3 + 3x − a + 2.
If both x + 1 and x − 1 are factors of ax3 + x2 − 2x + b, find the values of a and b.
x3 − 6x2 + 3x + 10
If x − a is a factor of x3 −3x2a + 2a2x + b, then the value of b is
If x − 3 is a factor of x2 − ax − 15, then a =
If x51 + 51 is divided by x + 1, the remainder is
