Advertisements
Advertisements
प्रश्न
Factorize: a (a + b)3 - 3a2b (a + b)
उत्तर
Taking (a + b) common in two terms
= (a + b){a (a + b)2 - 3a2b}
Now, using (a + b)2 = a2 + b2 + 2ab
= (a + b){a (a2 + b2 + 2ab) - 3a2b}
= (a + b){a3 + ab2 + 2a2b - 3a2b}
= (a + b){a3 + ab2 - a2b}
= (a + b) a{a2 + b2 - ab}
= a (a + b)(a2 + b2 - ab)
∴ a (a + b)3 - 3a2b (a + b) = a (a + b)(a2 + b2 - ab)
APPEARS IN
संबंधित प्रश्न
Factorize `2x^2 + 3sqrt5x + 5`
Factorize `9(2a - b)^2 - 4(2a - b) - 13`
Factorize the following expressions:
1029 – 3x3
27x3 − y3 − z3 − 9xyz
The value of \[\frac{(2 . 3 )^3 - 0 . 027}{(2 . 3 )^2 + 0 . 69 + 0 . 09}\]
Divide: 6x2 + 5x - 6 by 2x + 3
Express the following as an algebraic expression:
The product of x and y divided by m.
Express the following as an algebraic expression:
The product of 12, x, y and z minus the product of 5, m and n.
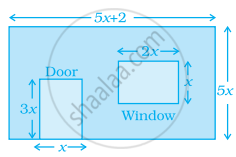
The figure shows the dimensions of a wall having a window and a door of a room. Write an algebraic expression for the area of the wall to be painted.
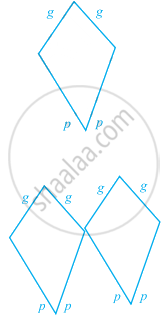
In the given figure, the length of a green side is given by g and that of the red side is given by p.

Write an expression for the following pattern. Also write an expression if 100 such shapes are joined together.