Question

Find area of the ellipse `x^2/5^2 + y^2/4^2` = 1

Answer 1

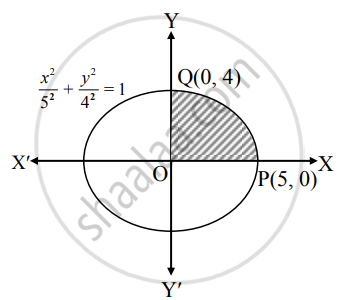
By the symmetry of the ellipse, required area of the ellipse is 4 times the area of the region OPQO.

For the region OPQO, the limits of integration are x = 0 and x = 5.

Given equation of the ellipse is `x^2/5^2 + y^2/4^2` = 1

∴ `y^2/4^2 = 1 - x^2/5^2`

∴ y² = `4^2(1 - x^2/5^2) = 4^2/5^2(5^2 - x^2)`

∴ y = `+- 4/5 sqrt(5^2 - x^2)`

∴ y = `4/5 sqrt(5^2 - x^2)`     ......[∵ In first quadrant, y > 0]

∴ Required area = 4(area of the region OPQO)

∴ `4 int_0^5 y  "d"x = 4int_0^5 4/5 sqrt(5^2 - x^2)  "d"x`

= `(4 xx 4)/5 int_0^5 sqrt((5)^2 - x^2)  "d"x`

= `16/5[x/2 sqrt((5)^2 - x^2) + (5)^2/2 sin^-1 (x/5)]_0^5`

= `16/5 {[5/2 sqrt((5)^2 - (5)^2) + (5)^2/2 sin^-1 (5/5)] - [0/2 sqrt((5)^2 - 0^2) + (5)^2/2 sin^-1 (0/5)]}`

= `16/5 [0 + 25/2 sin^-1 (1) - (0 + 0)]`

= `16/5 (25/2 xx pi/2)`

= 20π sq.units