Advertisements
Advertisements
प्रश्न
Find by integration, the volume of the solid generated by revolving about the x-axis, the region enclosed by y = 2x2, y = 0 and x = 1
उत्तर
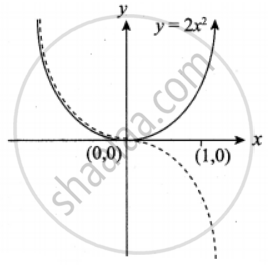
V = `int_"a"^"b" piy^2 "d"x`
= `int_0^1 pi(2x^2)^2 "d"x`
= `4pi int_0^1 x^4 "d"x`
V = `4pi[x^5/5]_0^1`
= `(4pi)/5` cubic units.
APPEARS IN
संबंधित प्रश्न
Find, by integration, the volume of the solid generated by revolving about the x axis, the region enclosed by y = e-2x, y = 0, x = 0 and x = 1
Find, by integration, the volume of the solid generated by revolving about the y axis, the region enclosed by x2 = 1 + y and y = 3
The region enclosed between the graphs of y = x and y = x2 is denoted by R. Find the volume generated when R is rotated through 360° about x-axis
Find, by integration, the volume of the container which is in the shape of a right circular conical frustum as shown in the Fig 9.46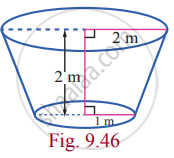
A watermelon has an ellipsoid shape which can be obtained by revolving an ellipse with major-axis 20 cm and minor-axis 10 cm about its major-axis. Find its volume using integration
Choose the correct alternative:
The volume of solid of revolution of the region bounded by y2 = x(a – x) about the x-axis is
