Advertisements
Advertisements
प्रश्न
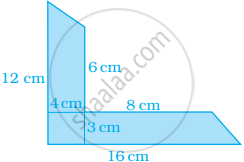
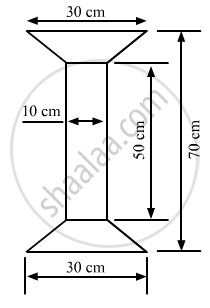
Find the area of Fig. as the sum of the areas of two trapezium and a rectangle.
Advertisements
उत्तर
In the given figure, we have a rectangle of length 50 cm and width 10 cm, and two similar trapeziums with parallel sides as 30 cm and 10 cm at both ends.
Suppose x is the perpendicular distance between the parallel sides in both the trapeziums.
We have:
Total length of the given figure = Length of the rectangle + 2 x Perpendicular distancebetween the parallel sides in both the trapeziums
\[70 = 50 + 2 \times x\]
\[2\times x=70-50=20\]
\[x=\frac{20}{2}=10 cm\]
Now, area of the complete figure = (area of the rectangle with sides 50 cm and 10 cm) + 2 x (area of the trapezium with parallel sides 30 cm and 10 cm, and height 10 cm
\[=(50\times10)+2\times[\frac{1}{2}\times(30+10)\times(10)]\]
\[ = 500 + 2 \times [200]\]
= 900 cm2
संबंधित प्रश्न
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 12 dm and 20 dm, altitude = 10 dm
Find the area of a trapezium whose parallel sides of lengths 10 cm and 15 cm are at a distance of 6 cm from each other. Calculate this area as the difference of the area of a rectangle and the sum of the areas of two triangles.
The cross-section of a canal is a trapezium in shape. If the canal is 10 m wide at the top 6 m wide at the bottom and the area of cross-section is 72 m2 determine its depth.
The area of a trapezium is 1586 cm2 and the distance between the parallel sides is 26 cm. If one of the parallel sides is 38 cm, find the other.
If the area of a trapezium is 28 cm2 and one of its parallel sides is 6 cm, find the other parallel side if its altitude is 4 cm.
There is a pentagonal shaped park as shown in Fig. 20.50. Jyoti and Kavita divided it in two different ways.
Find the area of this park using both ways. Can you suggest some another way of finding its areas?
The following figure shows the cross-section ABCD of a swimming pool which is a trapezium in shape.
If the width DC, of the swimming pool, is 6.4 m, depth (AD) at the shallow end is 80 cm and depth (BC) at the deepest end is 2.4 m, find its area of the cross-section.
The sunshade of a window is in the form of isosceles trapezium whose parallel sides are 81 cm and 64 cm and the distance between them is 6 cm. Find the cost of painting the surface at the rate of ₹ 2 per sq.cm
A window is in the form of trapezium whose parallel sides are 105 cm and 50 cm respectively and the distance between the parallel sides is 60 cm. Find the cost of the glass used to cover the window at the rate of ₹ 15 per 100 sq.cm
In a trapezium if the sum of the parallel sides is 10 cm and the area is 140 sq.cm, then the height is
