Advertisements
Advertisements
प्रश्न
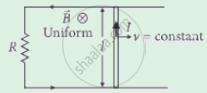
Find the current in the sliding rod AB (resistance = R) for the arrangement shown in figure. B is constant and is out of the paper. Parallel wires have no resistance. v is constant. Switch S is closed at time t = 0.

उत्तर
This is a similar problem as we discussed above. Here, a conductor of length d moves with speed v, perpendicular to the magnetic field B as shown in figure.
Due to this a motional emf is induced across two ends of rod (e = vBd). Since switch S is closed at time t = 0, capacitor is charged by this potential difference.
Let Q(t) be the charge on the capacitor and current flows from A to B.
Now, the induced current
`I = (dQ)/(dt) = (Bvd) - Q/(RC)`
`Q/(RC) + (dQ)/(dt) = (Bvd)/R`
`Q + RC (dQ)/(dt) = vBC` .....(Let `vBdC = A`)
`Q + RC Q/(dt) A`
`(dQ)/(A - Q) = 1/(RC) dt`
By integrating we have
`int_0^Q (dQ)/(A - Q) = 1/(RC) int_0^t dt - [In (A -Q) - In A] = t/(RC)`
In ``(A / Q)/A = - t/(RC)`
`(A - Q)/A = e^(-t/(RC))`
`Q = A(1 - e^(-t/(RC)))`
Current in the rod,
`I = (dQ)/(dt) = d/(dt) [A(1 - e^(-t/(RC)))]`
= `- A(e^-t/(RC))(- 1/(RC))`
`I = (vBd)/R e^(-t/(RC)`
APPEARS IN
संबंधित प्रश्न
A conducting disc of radius r rotates with a small but constant angular velocity ω about its axis. A uniform magnetic field B exists parallel to the axis of rotation. Find the motional emf between the centre and the periphery of the disc.
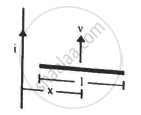
Figure shows a straight, long wire carrying a current i and a rod of length l coplanar with the wire and perpendicular to it. The rod moves with a constant velocity v in a direction parallel to the wire. The distance of the wire from the centre of the rod is x. Find the motional emf induced in the rod.
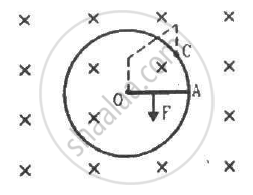
Consider the situation shown in the figure. Suppose the wire connecting O and C has zero resistance but the circular loop has a resistance Runiformly distributed along its length. The rod OA is made to rotate with a uniform angular speed ω as shown in the figure. Find the current in the rod when ∠ AOC = 90°.
A cycle wheel of radius 0.6 m is rotated with constant angular velocity of 15 rad/s in a region of magnetic field of 0.2 T which is perpendicular to the plane of the wheel. The e.m.f generated between its center and the rim is, ____________.
The emf induced across the ends of a conductor due to its motion in a magnetic field is called motional emf. It is produced due to magnetic Lorentz force acting on the free electrons of the conductor. For a circuit shown in the figure, if a conductor of length l moves with velocity v in a magnetic field B perpendicular to both its length and the direction of the magnetic field, then all the induced parameters are possible in the circuit.
Direction of current induced in a wire moving in a magnetic field is found using ______.
Motional e.m.f is the induced e.m.f. ______
Find the current in the wire for the configuration shown in figure. Wire PQ has negligible resistance. B, the magnetic field is coming out of the paper. θ is a fixed angle made by PQ travelling smoothly over two conducting parallel wires separated by a distance d.

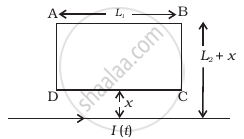
A rectangular loop of wire ABCD is kept close to an infinitely long wire carrying a current I(t) = Io (1 – t/T) for 0 ≤ t ≤ T and I(0) = 0 for t > T (Figure). Find the total charge passing through a given point in the loop, in time T. The resistance of the loop is R.
A rod of mass m and resistance R slides smoothly over two parallel perfectly conducting wires kept sloping at an angle θ with respect to the horizontal (Figure). The circuit is closed through a perfect conductor at the top. There is a constant magnetic field B along the vertical direction. If the rod is initially at rest, find the velocity of the rod as a function of time.

