Advertisements
Advertisements
प्रश्न
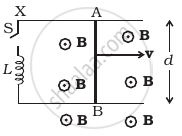
Find the current in the sliding rod AB (resistance = R) for the arrangement shown in figure. B is constant and is out of the paper. Parallel wires have no resistance. v is constant. Switch S is closed at time t = 0.
उत्तर
This is a similar problem as we discussed above. Here, a conductor of length d moves with speed v, perpendicular to magnetic field B as shown in figure.
Due to this, an motional emf is induced across two ends of rod (e = vBd).
Since, switch S is closed at time t = 0 current starts growing in the inductor by the potential difference due to motional emf.
By applying KVL in the given circuit, we have
`- L (dI)/(dt) + vBd = IR` or `L (dI)/(dt) + IR = vBd`
This is the linear differential equation.
On solving, we get
`I = (vBd)/R + Ae^((- Rt)/2)`
At t = 0, I = 0
⇒ `A = - (vBd)/R`
⇒ `I = (vBd)/R (1 - e^((- Rt)/L))`
This is the required expression of current.
APPEARS IN
संबंधित प्रश्न
A 20 cm long conducting rod is set into pure translation with a uniform velocity of 10 cm s−1 perpendicular to its length. A uniform magnetic field of magnitude 0.10 T exists in a direction perpendicular to the plane of motion. (a) Find the average magnetic force on the free electrons of the rod. (b) For what electric field inside the rod, the electric force on a free elctron will balance the magnetic force? How is this electric field created? (c) Find the motional emf between the ends of the rod.
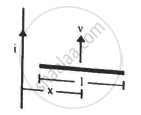
Figure shows a straight, long wire carrying a current i and a rod of length l coplanar with the wire and perpendicular to it. The rod moves with a constant velocity v in a direction parallel to the wire. The distance of the wire from the centre of the rod is x. Find the motional emf induced in the rod.
A wire of length 50 cm moves with a velocity of 300 m/min, perpendicular to a magnetic field. If the e.m.f. induced in the wire is 2 V, the magnitude of the field in tesla is ______.
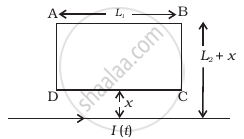
A rectangular loop of wire ABCD is kept close to an infinitely long wire carrying a current I(t) = Io (1 – t/T) for 0 ≤ t ≤ T and I(0) = 0 for t > T (Figure). Find the total charge passing through a given point in the loop, in time T. The resistance of the loop is R.
Find the current in the sliding rod AB (resistance = R) for the arrangement shown in figure. B is constant and is out of the paper. Parallel wires have no resistance. v is constant. Switch S is closed at time t = 0.

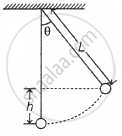
A simple pendulum with a bob of mass m and conducting wire of length L swings under gravity through an angle θ. The component of the earth's magnetic field in the direction perpendicular to the swing is B. Maximum emf induced across the pendulum is ______.
(g = acceleration due to gravity)
A wire 5 m long is supported horizontally at a height of 15 m along an east-west direction. When it is about to hit the ground, calculate the average e.m.f. induced in it. (g = 10 m/s2)
Derive an expression for the total emf induced in a conducting rotating rod.
A magnetic flux associated with a coil changes by 0.04 Wb in 0.2 second. The induced emf with coil is ______.
An aircraft of wing span of 60 m flies horizontally in earth’s magnetic field of 6 × 10−5 T at a speed of 500 m/s. Calculate the e.m.f. induced between the tips of the wings of the aircraft.
