Advertisements
Advertisements
प्रश्न
Find the value of the unknown x in the following diagram:
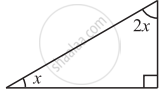
उत्तर
The sum of all interior angles of a triangle is 180°. By using this property, these problems can be solved as follows:
x + 2x + 90° = 180°
3x = 180° − 90°
3x = 90°
x = `(90°)/3`
x = 30°
APPEARS IN
संबंधित प्रश्न
In the given figure find m∠P.
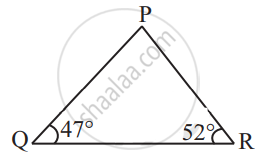
In the following triangle, find the value of x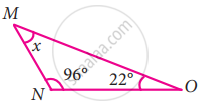
The angles of a triangle are in the ratio 2 : 3 : 4. Find the angles of the triangle.
In a triangle, one angle is of 90°. Then ______.
- The other two angles are of 45° each
- In remaining two angles, one angle is 90° and other is 45°
- Remaining two angles are complementary
In the given option(s) which is true?
If two angles of a triangle are 60° each, then the triangle is ______.
In ∆PQR, if PQ = QR and ∠Q = 100°, then ∠R is equal to ______.
It is possible to have a triangle in which each angle is greater than 60°.
In a triangle ABC, the measure of angle A is 40° less than the measure of angle B and 50° less than that of angle C. Find the measure of ∠A.
In ΔPQR, if 3∠P = 4∠Q = 6∠R, calculate the angles of the triangle.
In ΔDEF, ∠D = 60°, ∠E = 70° and the bisectors of ∠E and ∠F meet at O. Find (i) ∠F (i) ∠EOF.
