Advertisements
Advertisements
प्रश्न
In a triangle ABC, the measure of angle A is 40° less than the measure of angle B and 50° less than that of angle C. Find the measure of ∠A.
उत्तर
According to the question,
Measure of ∠A = ∠B = 40°
Measure of ∠C = ∠B – 40° + 50°
We know that, the sum of all three angles in a triangle is equal to 180°
i.e. ∠A + ∠B + = 180°°
⇒ (∠B – 40°) + ∠B + (∠B – 40° + 50°) = 180°
⇒ 3∠B – 30° = 180°
⇒ 3∠B = 210°
∴ ∠B = `210^circ/3` = 70°
So, the measure of ∠A = 70° – 40° = 30°.
APPEARS IN
संबंधित प्रश्न
Find the value of the unknown x in the following diagram:
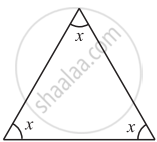
Find the value of the unknown x in the following diagram:
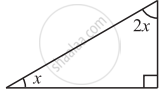
In the following triangle, find the value of x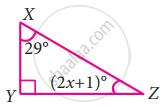
In the following triangle, find the value of x
In the following triangle, find the value of x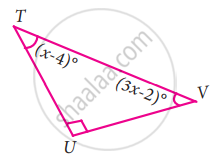
Observe the figure and find the value of ∠A + ∠N + ∠G + ∠L + ∠E + ∠S
In ∆RST, ∠S is 10° greater than ∠R and ∠T is 5° less than ∠S, find the three angles of the triangle
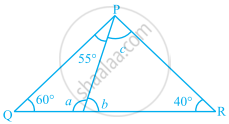
In the given figure, find the values of a, b and c
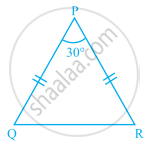
In ΔPQR of the given figure, PQ = PR. Find the measures of ∠Q and ∠R.
In ΔPQR, if 3∠P = 4∠Q = 6∠R, calculate the angles of the triangle.
