Advertisements
Advertisements
प्रश्न
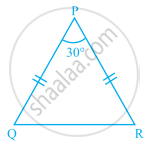
In ΔPQR of the given figure, PQ = PR. Find the measures of ∠Q and ∠R.
उत्तर
∠PRQ ∠PQR ......[∵ PQ = PR (Given)]
Now, In ∆POR
∠PQR + ∠PRQ + ∠QPR = 180° ......[Angle sum property]
⇒ 2∠POR + 30° = 180° .....[Using (1)]
⇒ 2∠PQR = 180° – 30° = 150°
⇒ ∠PQR = `150^circ/2` = 75°
Thus, ∠Q = ∠R = 75°
APPEARS IN
संबंधित प्रश्न
In the given figure, the side QR of ΔPQR is produced to a point S. If the bisectors of ∠PQR and ∠PRS meet at point T, then prove that ∠QTR = 1/2∠QPR.

Find the value of the unknown x in the given diagram:
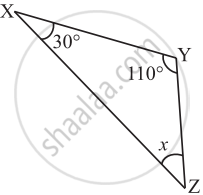
Find the value of the unknown x in the following diagram:
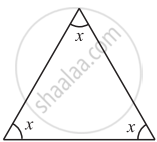
In the following triangle, find the value of x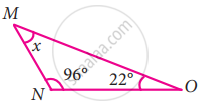
In ∆RST, ∠S is 10° greater than ∠R and ∠T is 5° less than ∠S, find the three angles of the triangle
In ∆ABC, if ∠B is 3 times ∠A and ∠C is 2 times ∠A, then find the angle
Angles of a triangle are in the ratio 2 : 4 : 3. The smallest angle of the triangle is ______.
A triangle ABC is right angled at A. L is a point on BC such that AL ⊥ BC. Prove that ∠BAL = ∠ACB.
In a triangle ABC, the measure of angle A is 40° less than the measure of angle B and 50° less than that of angle C. Find the measure of ∠A.
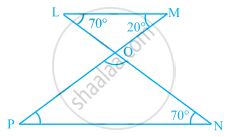
In the given figure, find the measures of ∠PON and ∠NPO.