Advertisements
Advertisements
प्रश्न
In ΔDEF, ∠D = 60°, ∠E = 70° and the bisectors of ∠E and ∠F meet at O. Find (i) ∠F (i) ∠EOF.
उत्तर
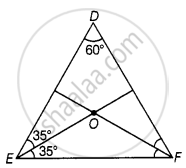
(i) As we know,
∠D + ∠E + ∠F = 180° ......[Angle sum property of a triangle]
⇒ 60° + 70° + ∠F = 180° ......[∵ ∠D = 60° and ∠E = 70°]
⇒ ∠F = 180° – 130°
⇒ ∠F = 50°
(ii) Now, as FO is the bisector of ∠F
So, ∠EFO = `(∠F)/2 = 50^circ/2` = 25°
And ∠OEF = `(∠E)/2 = 70^circ/2` = 35° ......[∵ ∠D = 60° and ∠E = 70°]
In ΔEOF, ∠EOF + ∠OEF + ∠OFE = 180° ......[Angle sum property of a triangle]
⇒ ∠EOF + 35° + 25° = 180°
⇒ ∠EOF = 180° – 60°
⇒ ∠EOF = 120°
APPEARS IN
संबंधित प्रश्न
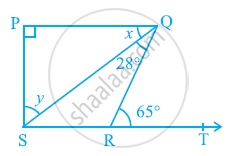
In the given figure, if PQ ⊥ PS, PQ || SR, ∠SQR = 28º and ∠QRT = 65º, then find the values of x and y.
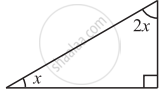
Find the value of the unknown x in the following diagram:
In a right angled triangle ABC, ∠B is right angle, ∠A is x + 1 and ∠C is 2x + 5. Find ∠A and ∠C
Can a triangle have all angles less than 60°? Give reason for your answer.
Bisectors of interior ∠B and exterior ∠ACD of a ∆ABC intersect at the point T. Prove that `∠BTC = 1/2 ∠BAC`.
Prove that through a given point, we can draw only one perpendicular to a given line.
[Hint: Use proof by contradiction].
In an isosceles triangle, one angle is 70°. The other two angles are of ______.
- 55° and 55°
- 70° and 40°
- any measure
In the given option(s) which of the above statement(s) are true?
If one angle of a triangle is equal to the sum of the other two angles, the triangle is ______.
If one of the angles of a triangle is 110°, then the angle between the bisectors of the other two angles is ______.
In a right-angled triangle if an angle measures 35°, then find the measure of the third angle.
