Advertisements
Advertisements
प्रश्न
In a right angled triangle ABC, ∠B is right angle, ∠A is x + 1 and ∠C is 2x + 5. Find ∠A and ∠C
उत्तर
Given in ∆ABC ∠B = 90°
∠A = x + 1
∠B = 2x + 5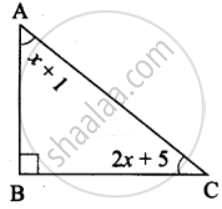
By angle sum property of triangle
Sum of three angles of ∆ABC = 180°
∠A + ∠B + ∠C = 180°
(x + 1) + 90° + (2x + 5) = 180°
x + 2x + 1° + 90° + 5° = 180°
3x + 96° = 180°
3x = 180° – 96° = 84°
x = `(84^circ)/3` = 28°
∠A = x + 1 = 28 + 1 = 29
∠C = 2x + 5
= 2(28) + 5
= 56 + 5
= 61
∴ ∠A = 29°
∠C = 61°
APPEARS IN
संबंधित प्रश्न
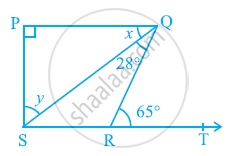
In the given figure, if PQ ⊥ PS, PQ || SR, ∠SQR = 28º and ∠QRT = 65º, then find the values of x and y.
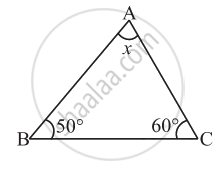
Find the value of the unknown x in the following diagram:
Find the value of the unknown x and y in the following diagram:

In a right angled triangle MNO, ∠N = 90°, MO is extended to P. If ∠NOP = 128°, find the other two angles of ∆MNO
In the given figure, which of the following statement is true?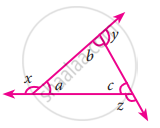
If one angle of a triangle is equal to the sum of the other two angles, then the triangle is ______.
How many triangles can be drawn having its angles as 53°, 64° and 63°? Give reason for your answer.
In the given figure, PQ = PS. The value of x is ______.

In triangle XYZ, the measure of angle X is 30° greater than the measure of angle Y and angle Z is a right angle. Find the measure of ∠Y.
In a right-angled triangle if an angle measures 35°, then find the measure of the third angle.
