Advertisements
Advertisements
प्रश्न
Find the values of k for which the points A(k + 1, 2k), B(3k, 2k + 3) and (5k – 1, 5k) are collinear.
उत्तर
A(k + 1, 2k) , B(3k, 2k + 3) and (5k – 1, 5k)
If 3 points are collinear then area of triangle formed by them = 0
`1/2[(k+1)(2k+3-5k)- 2k(3k-5k+1)+1(15k^2- 10k^2- 2k+ 15k - 3)= 0`
`1/2[-3k^2+3k-3k+3+4k^2-2k+15k^2-10k^2-2k+15k-3]=0`
`1/2[6k^2+11k]=0`
`6k^2+11k=0`
`k=0,-11/6`
APPEARS IN
संबंधित प्रश्न
In each of the following find the value of 'k', for which the points are collinear.
(7, -2), (5, 1), (3, -k)
ΔABC is isosceles with AB = AC = 7.5 cm and BC = 9 cm (see the given figure). The height AD from A to BC, is 6 cm. Find the area of ΔABC. What will be the height from C to AB i.e., CE?

Find the area of a triangle whose vertices are
(a, c + a), (a, c) and (−a, c − a)
Prove that the points (2,3), (-4, -6) and (1, 3/2) do not form a triangle.
prove that the points A (7, 10), B(-2, 5) and C(3, -4) are the vertices of an isosceles right triangle.
Show that the points are the vertices of an isosceles right triangle.
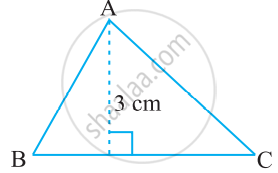
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
Points A(–6, 10), B(–4, 6) and C(3, –8) are collinear such that AB = `2/9` AC.
In the given figure, area of ΔPQR is 20 cm2 and area of ΔPQS is 44 cm2. Find the length RS, if PQ is perpendicular to QS and QR is 5 cm.

Find the missing value:
| Base | Height | Area of Triangle |
| 22 cm | ______ | 170.5 cm2 |
