Advertisements
Advertisements
प्रश्न
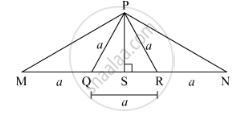
From the information given in the figure, prove that PM = PN =
उत्तर १
Since ∆PQR is an equilateral triangle, PS is the perpendicular bisector of QR.
∴ QS = SR =
Now, According to Pythagoras theorem,
In ∆PQS,
In ∆PMS,
In ∆PNS,
From (3) and (4), we get
PM = PN =
Hence, PM = PN =
उत्तर २
From figure,
In ∆PMR
MQ = QR = a ...(given)
∴ Q is a midpoint of MR.
∴ seg PQ is the median.
∴ According to Apollonius's theorem,
PM2 + PR2 = 2PQ2 + 2MQ2
∴ PM2 + a2 = 2a2 + 2a2
∴ PM2 + a2 = 4a2
∴ PM2 = 4a2 − a2
∴ PM2 = 3a2 ...Taking square root
PM =
From figure,
In ∆PNQ
NR = RQ = a ...(given)
∴ R is a midpoint of NQ.
∴ seg PR is the median.
∴ According to Apollonius's theorem,
PN2 + PQ2 = 2PR2 + 2NR2
∴ PN2 + a2 = 2a2 + 2a2
∴ PN2 + a2 = 4a2
∴ PN2 = 4a2 − a2
∴ PN = 3a2 ...Taking square root
PN =
From (3) and (4), we get
PM = PN =
∴ PM = PN =
APPEARS IN
संबंधित प्रश्न
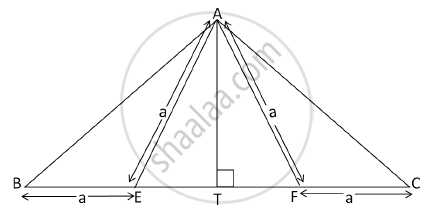
In the following figure, AE = EF = AF = BE = CF = a, AT ⊥ BC. Show that AB = AC =
Find the length of the side and perimeter of an equilateral triangle whose height is
∆ABC is an equilateral triangle. Point P is on base BC such that PC =
Find the length of the hypotenuse in a right angled triangle where the sum
of the squares of the sides making right angle is 169.
(A)15 (B) 13 (C) 5 (D) 12
Prove that, in a right angled triangle, the square of the hypotenuse is
equal to the sum of the squares of remaining two sides.
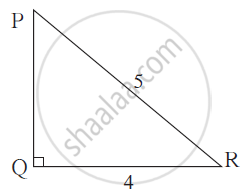
In right angled triangle PQR,
if ∠ Q = 90°, PR = 5,
QR = 4 then find PQ and hence find tan R.
Choose the correct alternative:
ΔABC and ΔDEF are equilateral triangles. If ar(ΔABC): ar(ΔDEF) = 1 : 2 and AB = 4, then what is the length of DE?
From given figure, In ∆ABC, AD ⊥ BC, then prove that AB2 + CD2 = BD2 + AC2 by completing activity.
Activity: From given figure, In ∆ACD, By pythagoras theorem
AC2 = AD2 +
∴ AD2 = AC2 – CD2 ......(I)
Also, In ∆ABD, by pythagoras theorem,
AB2 =
∴ AD2 = AB2 – BD2 ......(II)
∴
∴ AB2 + CD2 = AC2+ BD2
A ladder 10 m long reaches a window 8 m above the ground. Find the distance of the foot of the ladder from the base of wall. Complete the given activity.
Activity: As shown in figure suppose

PR is the length of ladder = 10 m
At P – window, At Q – base of wall, At R – foot of ladder
∴ PQ = 8 m
∴ QR = ?
In ∆PQR, m∠PQR = 90°
By Pythagoras Theorem,
∴ PQ2 +
Here, PR = 10, PQ =
From equation (I)
82 + QR2 = 102
QR2 = 102 – 82
QR2 = 100 – 64
QR2 =
QR = 6
∴ The distance of foot of the ladder from the base of wall is 6 m.
Complete the following activity to find the length of hypotenuse of right angled triangle, if sides of right angle are 9 cm and 12 cm.
Activity: In ∆PQR, m∠PQR = 90°
By Pythagoras Theorem,
PQ2 +
∴ PR2 = 92 + 122
∴ PR2 =
∴ PR2 =
∴ PR = 15
∴ Length of hypotenuse of triangle PQR is
From given figure, in ∆PQR, if ∠QPR = 90°, PM ⊥ QR, PM = 10, QM = 8, then for finding the value of QR, complete the following activity.

Activity: In ∆PQR, if ∠QPR = 90°, PM ⊥ QR, ......[Given]
In ∆PMQ, by Pythagoras Theorem,
∴ PM2 +
∴ PQ2 = 102 + 82
∴ PQ2 =
∴ PQ2 =
∴ PQ =
Here, ∆QPR ~ ∆QMP ~ ∆PMR
∴ ∆QMP ~ ∆PMR
∴
∴ PM2 = RM × QM
∴ 102 = RM × 8
RM =
And,
QR = QM + MR
QR =
Find the diagonal of a rectangle whose length is 16 cm and area is 192 sq.cm. Complete the following activity.
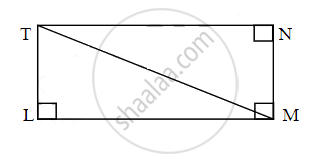
Activity: As shown in figure LMNT is a reactangle.
∴ Area of rectangle = length × breadth
∴ Area of rectangle =
∴ 192 =
∴ Breadth = 12 cm
Also,
∠TLM = 90° ......[Each angle of reactangle is right angle]
In ∆TLM,
By Pythagoras theorem
∴ TM2 = TL2 +
∴ TM2 = 122 +
∴ TM2 = 144 +
∴ TM2 = 400
∴ TM = 20
A congruent side of an isosceles right angled triangle is 7 cm, Find its perimeter
ΔPQR, is a right angled triangle with ∠Q = 90°, QR = b, and A(ΔPQR) = a. If QN ⊥ PR, then prove that QN =
