Advertisements
Advertisements
प्रश्न
Given A = (x + 2, -2) and B (11, 6). Find x if AB = 17.
उत्तर
AB = 17
AB2 = 289
(11 - x - 2)2 + (6 + 2)2 = 289
x2 + 81 - 18x + 64 = 289
x2 - 18x - 144 = 0
x2 - 24x + 6x - 144 = 0
x(x - 24) + 6(x - 24) = 0
(x - 24) (x + 6) = 0
x = 24, -6
APPEARS IN
संबंधित प्रश्न
If the point (x, y) is equidistant from the points (a + b, b – a) and (a – b, a + b), prove that bx = ay
Find the coordinates of the centre of the circle passing through the points (0, 0), (–2, 1) and (–3, 2). Also, find its radius.
If the distances of P(x, y) from A(5, 1) and B(–1, 5) are equal, then prove that 3x = 2y
Find the values of x, y if the distances of the point (x, y) from (-3, 0) as well as from (3, 0) are 4.
If A (-1, 3), B (1, -1) and C (5, 1) are the vertices of a triangle ABC, find the length of the median through A.
Find the distance between the points
(i) A(9,3) and B(15,11)
Find the distance of the following points from the origin:
(ii) B(-5,5)
Find the distance of the following points from the origin:
(iii) C (-4,-6)
`" Find the distance between the points" A ((-8)/5,2) and B (2/5,2)`
Find the distance of the following point from the origin :
(6 , 8)
x (1,2),Y (3, -4) and z (5,-6) are the vertices of a triangle . Find the circumcentre and the circumradius of the triangle.
Find the distance between the points (a, b) and (−a, −b).
Prove that the points P (0, -4), Q (6, 2), R (3, 5) and S (-3, -1) are the vertices of a rectangle PQRS.
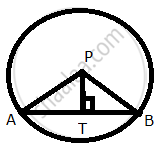
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
Calculate the distance between the points P (2, 2) and Q (5, 4) correct to three significant figures.
Find the distance of the following points from origin.
(5, 6)
If the point (x, y) is at equidistant from the point (a + b, b – a) and (a-b, a + b). Prove that ay = bx.
Find the value of a, if the distance between the points A(–3, –14) and B(a, –5) is 9 units.
If the point A(2, – 4) is equidistant from P(3, 8) and Q(–10, y), find the values of y. Also find distance PQ.
Show that points A(–1, –1), B(0, 1), C(1, 3) are collinear.
