Advertisements
Advertisements
प्रश्न
x (1,2),Y (3, -4) and z (5,-6) are the vertices of a triangle . Find the circumcentre and the circumradius of the triangle.
उत्तर
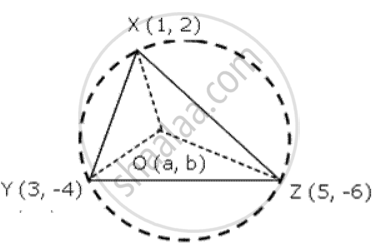
Circumcentre of Δ XYZ will pass through the vertices X , Y and Z
OX = OY (radi of same circle),
⇒ OX2 = OY2
(a - 1)2 + (b - 2)2 = (a - 3)2 + (b + 4)2
⇒ 1 - 2a + 4 - 4b = 9 - 6a + 16 + 8b
⇒ 4a - 12b = 20
⇒ a - 3b = 5 .........(1)
OY = OZ (radii of same circle)
OY2 = OZ2
(a - 3)2 + (b + 4)2 = (a - 5)2 + (b + 6)2
⇒ 9 - 6a + 16 + 8b = 25 - 10a + 36 + 12b
⇒ 4a - 4b = 36
⇒ a - b = 9 .........(2)
a - 3b = 5 ..........(1)
a - b = 9
- 2b = -4
b = 2
a = 11
Circumcentre of Δ XYZ is O (11 , 2)
Circumradius = `sqrt ((11 - 1)^2 + (2 - 2)^2) = sqrt 100` = 10 units
APPEARS IN
संबंधित प्रश्न
Show that the quadrilateral whose vertices are (2, −1), (3, 4) (−2, 3) and (−3,−2) is a rhombus.
If the point A(x,2) is equidistant form the points B(8,-2) and C(2,-2) , find the value of x. Also, find the value of x . Also, find the length of AB.
Prove that the points (4 , 6) , (- 1 , 5) , (- 2, 0) and (3 , 1) are the vertices of a rhombus.
Prove that the points (0 , 0) , (3 , 2) , (7 , 7) and (4 , 5) are the vertices of a parallelogram.
A(2, 5), B(-2, 4) and C(-2, 6) are the vertices of a triangle ABC. Prove that ABC is an isosceles triangle.
Find the distance of the following points from origin.
(5, 6)
KM is a straight line of 13 units If K has the coordinate (2, 5) and M has the coordinates (x, – 7) find the possible value of x.
Use distance formula to show that the points A(-1, 2), B(2, 5) and C(-5, -2) are collinear.
Using distance formula decide whether the points (4, 3), (5, 1), and (1, 9) are collinear or not.
Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
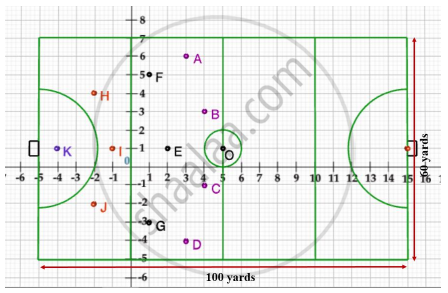
What are the coordinates of the position of a player Q such that his distance from K is twice his distance from E and K, Q and E are collinear?
