Advertisements
Advertisements
प्रश्न
Prove that the points (0 , 0) , (3 , 2) , (7 , 7) and (4 , 5) are the vertices of a parallelogram.
उत्तर
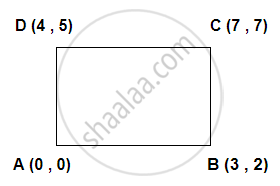
AB = `sqrt ((3 - 0)^2 + (2 - 0)^2) = sqrt (9 + 4) = sqrt 13` units
BC = `sqrt ((3 - 7)^2 + (2 - 7)^2) = sqrt (16 + 25) = sqrt 41` units
CD = `sqrt ((7 - 4)^2 + (7 - 5)^2) = sqrt (9 + 4) = sqrt 13` units
DA = `sqrt ((4 - 0)^2 + (5 - 0)^2) = sqrt (16 + 25) = sqrt 41` units
∵ AB = CD and BC = DA
∴ ABCD is a parallelogram.
APPEARS IN
संबंधित प्रश्न
Find the distance between two points
(i) P(–6, 7) and Q(–1, –5)
(ii) R(a + b, a – b) and S(a – b, –a – b)
(iii) `A(at_1^2,2at_1)" and " B(at_2^2,2at_2)`
Find the value of x, if the distance between the points (x, – 1) and (3, 2) is 5.
Find the values of x, y if the distances of the point (x, y) from (-3, 0) as well as from (3, 0) are 4.
Using the distance formula, show that the given points are collinear:
(-1, -1), (2, 3) and (8, 11)
Find the distance of the following point from the origin :
(5 , 12)
Find the coordinate of O , the centre of a circle passing through A (8 , 12) , B (11 , 3), and C (0 , 14). Also , find its radius.
Show that the points A (5, 6), B (1, 5), C (2, 1) and D (6, 2) are the vertices of a square ABCD.
Find the point on y-axis whose distances from the points A (6, 7) and B (4, -3) are in the ratio 1: 2.
Show that the point (11, – 2) is equidistant from (4, – 3) and (6, 3)
Name the type of triangle formed by the points A(–5, 6), B(–4, –2) and C(7, 5).
