Advertisements
Advertisements
प्रश्न
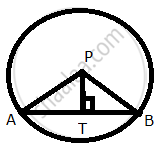
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
उत्तर
We know that the perpendicular from the center of a circle to a chord bisects the chord.
∴ AB = 2AT
= 2 x 12 units
= 24 units.
APPEARS IN
संबंधित प्रश्न
If A(4, 3), B(-1, y) and C(3, 4) are the vertices of a right triangle ABC, right-angled at A, then find the value of y.
Find the coordinates of the centre of the circle passing through the points (0, 0), (–2, 1) and (–3, 2). Also, find its radius.
Determine if the points (1, 5), (2, 3) and (−2, −11) are collinear.
Find the values of x, y if the distances of the point (x, y) from (-3, 0) as well as from (3, 0) are 4.
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Find the distance between the points
(i) A(9,3) and B(15,11)
Find the distance between the points
(ii) A(7,-4)and B(-5,1)
Find the distance between the points
A(1,-3) and B(4,-6)
Find the distance of a point (7 , 5) from another point on the x - axis whose abscissa is -5.
A(-2, -3), B(-1, 0) and C(7, -6) are the vertices of a triangle. Find the circumcentre and the circumradius of the triangle.
Prove that the points (0,3) , (4,3) and `(2, 3+2sqrt 3)` are the vertices of an equilateral triangle.
Find the point on y-axis whose distances from the points A (6, 7) and B (4, -3) are in the ratio 1: 2.
The distances of point P (x, y) from the points A (1, - 3) and B (- 2, 2) are in the ratio 2: 3.
Show that: 5x2 + 5y2 - 34x + 70y + 58 = 0.
Show that the point (0, 9) is equidistant from the points (– 4, 1) and (4, 1)
The distance between the points A(0, 6) and B(0, -2) is ______.
Point P(0, 2) is the point of intersection of y-axis and perpendicular bisector of line segment joining the points A(–1, 1) and B(3, 3).
Name the type of triangle formed by the points A(–5, 6), B(–4, –2) and C(7, 5).
If the point A(2, – 4) is equidistant from P(3, 8) and Q(–10, y), find the values of y. Also find distance PQ.
The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, – 9) and has diameter `10sqrt(2)` units.
Show that points A(–1, –1), B(0, 1), C(1, 3) are collinear.
