Advertisements
Advertisements
प्रश्न
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
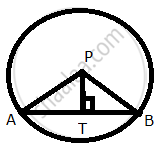
उत्तर
We know that the perpendicular from the center of a circle to a chord bisects the chord.
∴ AB = 2AT
= 2 x 12 units
= 24 units.
APPEARS IN
संबंधित प्रश्न
If P and Q are two points whose coordinates are (at2 ,2at) and (a/t2 , 2a/t) respectively and S is the point (a, 0). Show that `\frac{1}{SP}+\frac{1}{SQ}` is independent of t.
If P (2, – 1), Q(3, 4), R(–2, 3) and S(–3, –2) be four points in a plane, show that PQRS is a rhombus but not a square. Find the area of the rhombus
Name the type of quadrilateral formed, if any, by the following points, and give reasons for your answer:
(- 1, - 2), (1, 0), (- 1, 2), (- 3, 0)
Find the distance between the following pair of points:
(asinα, −bcosα) and (−acos α, bsin α)
If A (-1, 3), B (1, -1) and C (5, 1) are the vertices of a triangle ABC, find the length of the median through A.
Find the distance of the following points from the origin:
(i) A(5,- 12)
The long and short hands of a clock are 6 cm and 4 cm long respectively. Find the sum of the distances travelled by their tips in 24 hours. (Use π = 3.14) ?
Determine whether the points are collinear.
L(–2, 3), M(1, –3), N(5, 4)
The perimeter of a triangle with vertices (0, 4), (0, 0) and (3, 0) is ______.
P and Q are two points lying on the x - axis and the y-axis respectively . Find the coordinates of P and Q if the difference between the abscissa of P and the ordinates of Q is 1 and PQ is 5 units.
Prove that the points (4 , 6) , (- 1 , 5) , (- 2, 0) and (3 , 1) are the vertices of a rhombus.
Prove that the points (0 , 0) , (3 , 2) , (7 , 7) and (4 , 5) are the vertices of a parallelogram.
Find the distance between the following pairs of points:
(-3, 6) and (2, -6)
A point P lies on the x-axis and another point Q lies on the y-axis.
Write the abscissa of point Q.
If the length of the segment joining point L(x, 7) and point M(1, 15) is 10 cm, then the value of x is ______
Find distance CD where C(– 3a, a), D(a, – 2a)
The distance between the points A(0, 6) and B(0, -2) is ______.
The centre of a circle is (2a, a – 7). Find the values of a if the circle passes through the point (11, – 9) and has diameter `10sqrt(2)` units.
The distance between the points (0, 5) and (–3, 1) is ______.
