Advertisements
Advertisements
प्रश्न
If ABCD is a parallelogram, then prove that
𝑎𝑟 (Δ𝐴𝐵𝐷) = 𝑎𝑟 (Δ𝐵𝐶𝐷) = 𝑎𝑟 (Δ𝐴𝐵𝐶) = 𝑎𝑟 (Δ𝐴𝐶𝐷) = `1/2` 𝑎𝑟 (||𝑔𝑚 𝐴𝐵𝐶𝐷) .
Advertisements
उत्तर
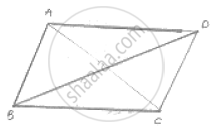
Given: ABCDis a parallelogram
To prove : area (Δ𝐴𝐵𝐷) = 𝑎𝑟 (ΔA𝐵𝐶) = are (Δ ACD)
= `1/2` 𝑎𝑟 (||𝑔𝑚 𝐴𝐵𝐶𝐷)
Proof: we know that diagonals of a parallelogram divides it into two equilaterals.
Since, AC is the diagonal.
Then, 𝑎𝑟 (Δ𝐴𝐵𝐶) = (Δ ACD) = `1/2` 𝑎𝑟 (||𝑔𝑚 𝐴𝐵𝐶𝐷)............ (1)
Since, BD is the diagonal
Then, 𝑎𝑟 (Δ𝐴𝐵𝐶) = 𝑎𝑟 (Δ𝐵𝐶𝐷) = `1/2` 𝑎𝑟 (||𝑔𝑚 𝐴𝐵𝐶𝐷)............ (2)
Compare equation (1) and (2)
∴ 𝑎𝑟 (Δ𝐴𝐵𝐶) = 𝑎𝑟 (Δ𝐴𝐶𝐷)
= 𝑎𝑟 (Δ𝐴𝐵𝐷) = 𝑎𝑟 (Δ𝐵𝐶𝐷) = `1/2` 𝑎𝑟 (||𝑔𝑚 𝐴𝐵𝐶𝐷)
APPEARS IN
संबंधित प्रश्न
In fig below, ABCD is a parallelogram, AE ⊥ DC and CF ⊥ AD. If AB = 16 cm, AE = 8
cm and CF = 10 cm, find AD.
A point D is taken on the side BC of a ΔABC such that BD = 2DC. Prove that ar(Δ ABD) =
2ar (ΔADC).
ABCD is a parallelogram whose diagonals AC and BD intersect at O. A line through O
intersects AB at P and DC at Q. Prove that ar (Δ POA) = ar (Δ QOC).
In square ABCD, P and Q are mid-point of AB and CD respectively. If AB = 8cm and PQand BD intersect at O, then find area of ΔOPB.
ABCD is a parallelogram. P is the mid-point of AB. BD and CP intersect at Q such that CQ: QP = 3.1. If ar (ΔPBQ) = 10cm2, find the area of parallelogram ABCD.
The table given below contains some measures of the rectangle. Find the unknown values.
| Length | Breadth | Perimeter | Area |
| 13 cm | ? | 54 cm | ? |
What is the area of the rectangle? ________ square cm
Cheggu’s wife asked him to make a circle with the wire. She knew it had an area of 800 square meters.
- Why did Cheggu not choose a rectangle? Explain.
Find the area of the following figure by counting squares:
Find the area of the following figure by counting squares:
