Question

Let ABC be a triangle of area 24 sq. units and PQR be the triangle formed by the mid-points of the sides of Δ ABC. Then the area of ΔPQR is

विकल्प

• 12 sq. units

•  6 sq. units

• 4 sq. units

• 3 sq. units

Answer 1

Given: (1) The Area of ΔABC = 24 sq units.

(2) ΔPQR is formed by joining the midpoints of ΔABC

To find: The area of ΔPQR

Calculation: In ΔABC, we have

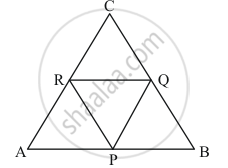
Since Q and R are the midpoints of BC and AC respectively.

∴  PQ || BA ⇒  PQ || BP

Similarly, RQ || BP. So BQRP is a parallelogram.

Similarly APRQ and PQCR are parallelograms.

We know that diagonal of a parallelogram bisect the parallelogram into two triangles of equal area.

Now, PR is a diagonal of ||^gmAPQR.

∴ Area of ΔAPR = Area of ΔPQR ……(1)

Similarly,

PQ is a diagonal of ||^gm PBQR

∴ Area of ΔPQR = Area of ΔPBQ ……(2)

QR is the diagonal of ||^gm  PQCR

∴ Area of ΔPQR = Area of ΔRCQ ……(3)

From (1), (2), (3) we have

Area of ΔAPR = Area of ΔPQR = Area of ΔPBQ = Area of ΔRCQ

But

Area of ΔAPR + Area of ΔPQR + Area of ΔPBQ + Area of ΔRCQ = Area of ΔABC

4(Area of ΔPBQ) = Area of ΔABC

`= 1/4 `Area of ΔABC 

 

∴ Area of ΔPBQ  `= 1/4 (24)`

= 6 sq units