Advertisements
Advertisements
प्रश्न
If α and β are the zeroes of the polynomial x2 – 1, then the value of (α + β) is ______.
विकल्प
2
1
–1
0
उत्तर
If α and β are the zeroes of the polynomial x2 – 1, then the value of (α + β) is 0.
Explanation:
Sum of zeroes of the quadratic equation
ax2 + bx + c = 0 is `(-b)/a`
∴ Sum of zeroes of x2 – 1 = x2 + 0x – 1 = 0 is `(-0)/1` = 0
∴ α + β = 0
APPEARS IN
संबंधित प्रश्न
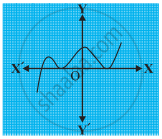
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
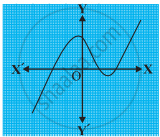
The graphs of y = p(x) are given in following figure, for some polynomials p(x). Find the number of zeroes of p(x).
If one zero of the polynomial `x^2-4x+1 is (2+sqrt3)` , write the other zero.
Find the sum of the zeros and the product of zeros of a quadratic polynomial, are `−1/2` and \ -3 respectively. Write the polynomial.
Find the zeroes of the quadratic polynomial `f(x) = 6x^2 – 3.`
Find the zeroes of the quadratic polynomial `f(x) = 4sqrt3x^2 + 5x – 2sqrt3`.
A quadratic polynomial, whose zeroes are -3 and 4, is ______.
Given that one of the zeroes of the cubic polynomial ax3 + bx2 + cx + d is zero, the product of the other two zeroes is ______.
A polynomial of degree n has ______.
If the graph of a polynomial intersects the x-axis at exactly two points, it need not be a quadratic polynomial.
