Advertisements
Advertisements
प्रश्न
If the angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13, then find the measure of the smallest angle.
उत्तर
We have, ∠A : ∠B : ∠C :∠D = 3 : 5 : 9 : 13 .
So, let ∠A = 3x,
∠B = 5x,
∠C = 9x
and ∠D = 13x
By angle sum property of a quadrilateral, we get:
∠A + ∠B + ∠C + ∠D = 360
3x + 5x + 9x + 13x = 360
30x = 360
`x =(360)/(30) `
x = 12
Smallest angle is :
∠A = 3x
∠A = 3(12°)
∠A = 36°
Hence, the smallest angle measures 36°.
APPEARS IN
संबंधित प्रश्न
Find the angle measure x in the given Figure
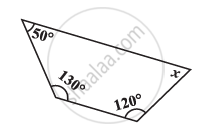
Find the angle measure x in the given Figure
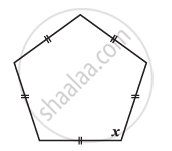

Find x + y + z
Find x in the following figures.

Two opposite angles of a parallelogram are (3x – 2)° and (50 – x)°. Find the measure of each angle of the parallelogram .
PQRS is a square such that PR and SQ intersect at O. State the measure of ∠POQ.
In a quadrilateral ABCD, bisectors of angles A and B intersect at O such that ∠AOB = 75°, then write the value of ∠C + ∠D.
In the given figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.
In a rhombus ABCD, if ∠ACB = 40°, then ∠ADB =
Can all the four angles of a quadrilateral be obtuse angles? Give reason for your answer.
