Question

If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a ______.

विकल्प

• rectangle

• rhombus

• parallelogram

• quadrilateral whose opposite angles are supplementary

Answer 1

If bisectors of ∠A and ∠B of a quadrilateral ABCD intersect each other at P, of ∠B and ∠C at Q, of ∠C and ∠D at R and of ∠D and ∠A at S, then PQRS is a quadrilateral whose opposite angles are supplementary

Explanation:

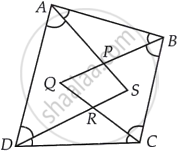
Sum of all angles of a quadrilateral is 360°

⇒ ∠A + ∠B + ∠C + ∠D = 360°

On dividing both sides by 2,

⇒ `1/2` (∠A + ∠B + ∠C + ∠D) = `1/2` × 360° = 180°

∵ AP, PB, RC and RD are bisectors of ∠A, ∠B, ∠C and ∠D

⇒ ∠PAB + ∠ABB + ∠RCD + ∠RDC = 180°  ...(1)

Sum of all angles of a triangle is 180°

∴ ∠PAB + ∠APB + ∠ABP = 180°

⇒ ∠PAB + ∠ABP = 180° – ∠APB  ...(2)

Similarly,

∴ ∠RDC + ∠RCD + ∠CRD = 180°

⇒ ∠RDC + ∠RCD = 180° – ∠CRD  ...(3)

Putting (2) and (3) in (1),

180° – ∠APB + 180° – ∠CRD = 180°

⇒ 360° – ∠APB – ∠CRD = 180°

⇒ ∠APB + ∠CRD = 360° – 180°

⇒ ∠APB + ∠CRD = 180°  ...(4)

Now,

∠SPQ = ∠APB   ...[Vertically opposite angles]

∠SRQ = ∠DRC  ...[Vertically opposite angles]

Putting in (4),

⇒ ∠SPQ + ∠SRQ = 180°