Advertisements
Advertisements
प्रश्न
If the points (2, 1) and (1, -2) are equidistant from the point (x, y), show that x + 3y = 0.
उत्तर
Let p(x, y), Q(2, 1), R(1, -2) be the given points
Here `x_1 = x`, `y_1 = y`
`x_2 = 2, y_2 = 1`
The distance between two points
p(x,y) and Q(2, 1) is given by
`PQ = sqrt((2- x)^2 + (1 - y)^2)`
Similarly
Now both these distance are given to be the same
PQ = PR
`sqrt((2- x)^2 + (1 - y)^2) = sqrt((1 - x)^2 + (-2 - y)^2)`
Squaring both the sides
`=> sqrt((2- x)^2 + (1 - y)^2) = sqrt((1 - x)^2 + (-2 - y))`
Squaring both the sides
`=> (2 - x)^2 + (1 - y)^2 = (1 - x)^2 + (-2 - y)^2`
`=> 4 + x^2 - 4x + 1 + y^2 - 2y = 1 + x^2- 2x + 4 + y^2 + 4y`
`=> 4 + x^2 - 4x + 1 + y^2 - 2y -1 - x^2 + 2x - 4 - y^2 - 4y = 0`
`=>-2x - 6y = 0`
`=> -2(x + 3y) = 0`
=> x + 3y = 0
Hence prove
APPEARS IN
संबंधित प्रश्न
The perimeter of a triangle with vertices (0, 4), (0, 0) and (3, 0) is ______.
In what ratio does the point P(−4, y) divides the line segment joining the points A(−6, 10) and B(3, −8)? Hence find the value of y.
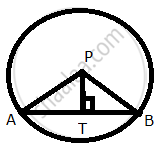
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
Calculate the distance between A (7, 3) and B on the x-axis whose abscissa is 11.
Give the relation that must exist between x and y so that (x, y) is equidistant from (6, -1) and (2, 3).
Show that P(– 2, 2), Q(2, 2) and R(2, 7) are vertices of a right angled triangle
A circle drawn with origin as the centre passes through `(13/2, 0)`. The point which does not lie in the interior of the circle is ______.
The points (– 4, 0), (4, 0), (0, 3) are the vertices of a ______.
If (– 4, 3) and (4, 3) are two vertices of an equilateral triangle, find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
Read the following passage:
|
Alia and Shagun are friends living on the same street in Patel Nagar. Shagun's house is at the intersection of one street with another street on which there is a library. They both study in the same school and that is not far from Shagun's house. Suppose the school is situated at the point O, i.e., the origin, Alia's house is at A. Shagun's house is at B and library is at C. |
Based on the above information, answer the following questions.
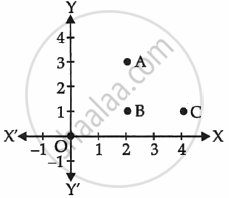
- How far is Alia's house from Shagun's house?
- How far is the library from Shagun's house?
- Show that for Shagun, school is farther compared to Alia's house and library.
OR
Show that Alia’s house, shagun’s house and library for an isosceles right triangle.
