Advertisements
Advertisements
प्रश्न
If (– 4, 3) and (4, 3) are two vertices of an equilateral triangle, find the coordinates of the third vertex, given that the origin lies in the interior of the triangle.
Advertisements
उत्तर
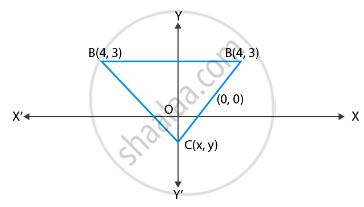
Let the vertices be (x, y)
Distance between (x, y) and (4, 3) is = `sqrt((x - 4)^2 + (y - 3)^2)` ...(1)
Distance between (x,y) and (– 4, 3) is = `sqrt((x + 4)^2 + (y - 3)^2)` ...(2)
Distance between (4, 3) and (– 4, 3) is = `sqrt((4 + 4)^2 + (3 - 3)^2) = sqrt(8)^2`= 8
According to the question,
Equation (1) = (2)
(x – 4)2 = (x + 4)2
x2 – 8x + 16 = x2 + 8x + 16
16x = 0
x = 0
Also, equation (1) = 8
(x – 4)2 + (y – 3)2 = 64 ...(3)
Substituting the value of x in (3)
Then (0 – 4)2 + (y – 3)2 = 64
(y – 3)2 = 64 – 16
(y – 3)2 = 48
y – 3 = `(+)4sqrt(3)`
y = `3(+) 4sqrt(3)`
Neglect y = `3(+) 4sqrt(3)` as if y = `3(+) 4sqrt(3)` then origin cannot interior of triangle
Therefore, the third vertex = `(0, 3 - 4sqrt(3))`
APPEARS IN
संबंधित प्रश्न
Show that the points (1, – 1), (5, 2) and (9, 5) are collinear.
If P (2, – 1), Q(3, 4), R(–2, 3) and S(–3, –2) be four points in a plane, show that PQRS is a rhombus but not a square. Find the area of the rhombus
Name the type of quadrilateral formed, if any, by the following point, and give reasons for your answer:
(4, 5), (7, 6), (4, 3), (1, 2)
Two opposite vertices of a square are (-1, 2) and (3, 2). Find the coordinates of other two
vertices.
The long and short hands of a clock are 6 cm and 4 cm long respectively. Find the sum of the distances travelled by their tips in 24 hours. (Use π = 3.14) ?
Find the distances between the following point.
P(–6, –3), Q(–1, 9)
The perimeter of a triangle with vertices (0, 4), (0, 0) and (3, 0) is ______.
Find the relation between a and b if the point P(a ,b) is equidistant from A (6,-1) and B (5 , 8).
Find the relation between x and y if the point M (x,y) is equidistant from R (0,9) and T (14 , 11).
The centre of a circle passing through P(8, 5) is (x+l , x-4). Find the coordinates of the centre if the diameter of the circle is 20 units.
A point P lies on the x-axis and another point Q lies on the y-axis.
If the abscissa of point P is -12 and the ordinate of point Q is -16; calculate the length of line segment PQ.
The points A (3, 0), B (a, -2) and C (4, -1) are the vertices of triangle ABC right angled at vertex A. Find the value of a.
Using distance formula decide whether the points (4, 3), (5, 1) and (1, 9) are collinear or not.
The equation of the perpendicular bisector of line segment joining points A(4,5) and B(-2,3) is ______.
A circle has its centre at the origin and a point P(5, 0) lies on it. The point Q(6, 8) lies outside the circle.
Find the points on the x-axis which are at a distance of `2sqrt(5)` from the point (7, – 4). How many such points are there?
The points A(–1, –2), B(4, 3), C(2, 5) and D(–3, 0) in that order form a rectangle.
Show that points A(–1, –1), B(0, 1), C(1, 3) are collinear.
|
Tharunya was thrilled to know that the football tournament is fixed with a monthly timeframe from 20th July to 20th August 2023 and for the first time in the FIFA Women’s World Cup’s history, two nations host in 10 venues. Her father felt that the game can be better understood if the position of players is represented as points on a coordinate plane. |
- At an instance, the midfielders and forward formed a parallelogram. Find the position of the central midfielder (D) if the position of other players who formed the parallelogram are :- A(1, 2), B(4, 3) and C(6, 6)
- Check if the Goal keeper G(–3, 5), Sweeper H(3, 1) and Wing-back K(0, 3) fall on a same straight line.
[or]
Check if the Full-back J(5, –3) and centre-back I(–4, 6) are equidistant from forward C(0, 1) and if C is the mid-point of IJ. - If Defensive midfielder A(1, 4), Attacking midfielder B(2, –3) and Striker E(a, b) lie on the same straight line and B is equidistant from A and E, find the position of E.
