Advertisements
Advertisements
प्रश्न
If PQRS is a square, then write the measure of ∠SRP.
उत्तर
The square PQRS is given as:
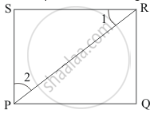
Since PQRS is a square.
Therefore,
PS = SR
and ∠PSR = 90°
Now, in ΔPSR, we have
PS = SR
That is, ∠1 = ∠2 (Angles opposite to equal sides are equal)
By angle sum property of a triangle.
∠PSR + ∠1 + ∠2 = 180°
∠PSR + 2∠1 = 180°
90° + 2∠1 = 180° (∠PSR = 90°)
2∠1 = 90°
∠1 = 45°
APPEARS IN
संबंधित प्रश्न
Three angles of a quadrilateral are respectively equal to 110°, 50° and 40°. Find its fourth angle
The angles of a quadrilateral are in the ratio 3 : 5 : 9 : 13 Find all the angles of the quadrilateral.
Find the measure of all the angles of a parallelogram, if one angle is 24° less than twice the smallest angle
ABCD is a parallelogram in which ∠A = 70°. Compute ∠B, ∠C and ∠D .
PQRS is a square such that PR and SQ intersect at O. State the measure of ∠POQ.
In the given figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.
If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
Can all the angles of a quadrilateral be right angles? Give reason for your answer.
The polygon in which sum of all exterior angles is equal to the sum of interior angles is called ______.
In a quadrilateral PQRS, ∠P = 50°, ∠Q = 50°, ∠R = 60°. Find ∠S. Is this quadrilateral convex or concave?
