Advertisements
Advertisements
प्रश्न
If the point P(2, 1) lies on the line segment joining points A(4, 2) and B(8, 4), then ______.
विकल्प
AP = \[\frac{1}{3}\text{AB}\]
AP = PB
PB = \[\frac{1}{3}\text{AB}\]
- AP = \[\frac{1}{2}\text{AB}\]
Advertisements
उत्तर
If the point P(2, 1) lies on the line segment joining points A(4, 2) and B(8, 4), then `underlinebb(AP = 1/2 AB)`.
Explanation:
Given that, the point P(2, 1) lies on the line segment joining the points A(4, 2) and B(8, 4), which shows in the figure below:
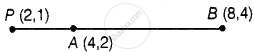
Now, distance between A(4, 2) and P(2, 1),
AP = `sqrt((x_2 - x_1)^2 + (y_2 - y_1)^2`
AP = `sqrt((2 - 4)^2 + (1 -2)^2`
= `sqrt((-2)^2 + (-1)^2`
= `sqrt(4 + 1)`
= `sqrt(5)`
Distance between A(4, 2) and B(8, 4),
AB = `sqrt((8 - 4)^2 + (4 - 2)^2`
= `sqrt((4)^2 + (2)^2`
= `sqrt(16 + 4)`
= `sqrt(20)`
= `2sqrt(5)`
Distance between B(8, 4) and P(2, 1),
BP = `sqrt((8 - 2)^2 + (4 - 1)^2`
= `sqrt(6^2 + 3^2`
= `sqrt(36 + 9)`
= `sqrt(45)`
= `3sqrt(5)`
∴ AB = `2sqrt(5)`
= 2AP
⇒ AP = `"AB"/2`
Hence, required condition is AP = `"AB"/2`
संबंधित प्रश्न
Find the distance between two points
(i) P(–6, 7) and Q(–1, –5)
(ii) R(a + b, a – b) and S(a – b, –a – b)
(iii) `A(at_1^2,2at_1)" and " B(at_2^2,2at_2)`
If P (2, – 1), Q(3, 4), R(–2, 3) and S(–3, –2) be four points in a plane, show that PQRS is a rhombus but not a square. Find the area of the rhombus
Find the distance between the points (0, 0) and (36, 15). Can you now find the distance between the two towns A and B discussed in Section 7.2.
In a classroom, 4 friends are seated at the points A, B, C and D as shown in the following figure. Champa and Chameli walk into the class and after observing for a few minutes, Champa asks Chameli, “Don’t you think ABCD is a square?” Chameli disagrees.
Using distance formula, find which of them is correct.
Find the distance between the following pair of points:
(a+b, b+c) and (a-b, c-b)
An equilateral triangle has two vertices at the points (3, 4) and (−2, 3), find the coordinates of the third vertex.
Two opposite vertices of a square are (-1, 2) and (3, 2). Find the coordinates of other two
vertices.
Find the distance between the points
A(-6,-4) and B(9,-12)
`" Find the distance between the points" A ((-8)/5,2) and B (2/5,2)`
Determine whether the points are collinear.
L(–2, 3), M(1, –3), N(5, 4)
Find the distance between the following point :
(p+q,p-q) and (p-q, p-q)
Find the distance between the following point :
(sec θ , tan θ) and (- tan θ , sec θ)
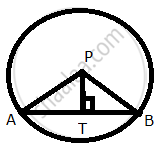
Point P (2, -7) is the centre of a circle with radius 13 unit, PT is perpendicular to chord AB and T = (-2, -4); calculate the length of AB.
Case Study -2
A hockey field is the playing surface for the game of hockey. Historically, the game was played on natural turf (grass) but nowadays it is predominantly played on an artificial turf.
It is rectangular in shape - 100 yards by 60 yards. Goals consist of two upright posts placed equidistant from the centre of the backline, joined at the top by a horizontal crossbar. The inner edges of the posts must be 3.66 metres (4 yards) apart, and the lower edge of the crossbar must be 2.14 metres (7 feet) above the ground.
Each team plays with 11 players on the field during the game including the goalie. Positions you might play include -
- Forward: As shown by players A, B, C and D.
- Midfielders: As shown by players E, F and G.
- Fullbacks: As shown by players H, I and J.
- Goalie: As shown by player K.
Using the picture of a hockey field below, answer the questions that follow:
What are the coordinates of the position of a player Q such that his distance from K is twice his distance from E and K, Q and E are collinear?
Find the value of a, if the distance between the points A(–3, –14) and B(a, –5) is 9 units.
Ayush starts walking from his house to office. Instead of going to the office directly, he goes to a bank first, from there to his daughter’s school and then reaches the office. What is the extra distance travelled by Ayush in reaching his office? (Assume that all distances covered are in straight lines). If the house is situated at (2, 4), bank at (5, 8), school at (13, 14) and office at (13, 26) and coordinates are in km.
|
Tharunya was thrilled to know that the football tournament is fixed with a monthly timeframe from 20th July to 20th August 2023 and for the first time in the FIFA Women’s World Cup’s history, two nations host in 10 venues. Her father felt that the game can be better understood if the position of players is represented as points on a coordinate plane. |
- At an instance, the midfielders and forward formed a parallelogram. Find the position of the central midfielder (D) if the position of other players who formed the parallelogram are :- A(1, 2), B(4, 3) and C(6, 6)
- Check if the Goal keeper G(–3, 5), Sweeper H(3, 1) and Wing-back K(0, 3) fall on a same straight line.
[or]
Check if the Full-back J(5, –3) and centre-back I(–4, 6) are equidistant from forward C(0, 1) and if C is the mid-point of IJ. - If Defensive midfielder A(1, 4), Attacking midfielder B(2, –3) and Striker E(a, b) lie on the same straight line and B is equidistant from A and E, find the position of E.
