Advertisements
Advertisements
प्रश्न
If two lines intersect, prove that the vertically opposite angles are equal.
उत्तर
Let us draw the figure.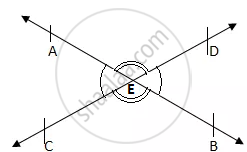
Here, we can see that
AB and CD intersect each other at point E.
The two pairs of vertically opposite angles are:
1st pair – ∠AEC and ∠BED
2nd pair – ∠AED and ∠BEC
We need to prove that the vertically opposite angles are equal, i.e.,
∠AEC = ∠BED and ∠AED = ∠BEC
Now, we can see that the ray AE stands on the line CD.
We know, if a ray stands on a line then the sum of the adjacent angles is equal to 180°.
⇒ ∠AEC + ∠AED = 180° (By linear pair axiom) ...(i)
Similarly, the ray DE stands on the line AEB.
⇒ ∠AED + ∠BED = 180° (By linear pair axiom) ...(ii)
From equations (i) and (ii), we have
∠AEC + ∠AED = ∠AED + ∠BED
⇒ ∠AEC = ∠BED ...(iii)
Similarly, the ray BE stands on the line CED.
⇒ ∠DEB + ∠CEB = 180° (By linear pair axiom) ...(iv)
Also, the ray CE stands on the line AEB.
⇒ ∠CEB + ∠AEC = 180° (By linear pair axiom) ...(v)
From equations (iv) and (v), we have
∠DEB + ∠CEB = ∠CEB + ∠AEC
⇒ ∠DEB = ∠AEC ...(vi)
Thus, from equation (iii) and equation (vi), we have
∠AEC = ∠BED and ∠DEB = ∠AEC
Therefore, it is proved that the vertically opposite angles are equal.
APPEARS IN
संबंधित प्रश्न
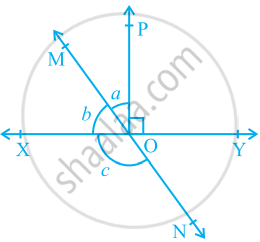
In the given figure, lines XY and MN intersect at O. If ∠POY = 90° and a : b = 2 : 3, find c.
Write the complement of the following angles .
30°
An angle is equal to 8 times its complement. Determine its measure.
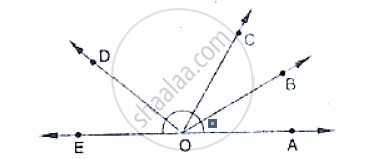
How many pairs of adjacent angles, in all, can you name in below fig.?
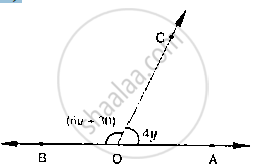
What value of y would make AOB a line in below fig, if ∠AOC = 4y and ∠BOC = (6y +
30)
statement are true and false
Angles forming a linear pair are supplementary.
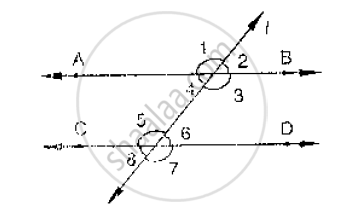
In below fig, AB CD and ∠1 and ∠2 are in the ratio 3 : 2. Determine all angles from 1 to 8.
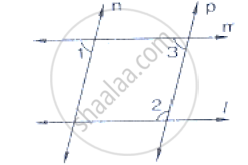
In the below fig, if l || m, n || p and ∠1 = 85°, find `∠`2.
Prove that if the two arms of an angle are perpendicular to the two arms of another angle, then the angles are either equal or supplementary
In the given figure, if AB || HF and DE || FG, then the measure of ∠FDE is