Advertisements
Advertisements
प्रश्न
In ΔABC, if bisectors of ∠ABC and ∠ACB intersect at O at angle of 120°, then find the measure of ∠A.
उत्तर
In the given ΔABC, ∠ABC = ∠ACB, the bisectors of ∠ABC and ∠ACBmeet at O and ∠BOC = 120°
We need to find the measure of ∠A
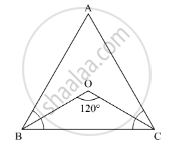
So here, using the corollary, “if the bisectors of ∠ABCand ∠ACBof a ΔABCmeet at a point O, then`∠BOC = 90° + 1/2 ∠A`
Thus, in ΔABC
`∠BOC = 90° + 1/2 ∠A`
` 120° = 90° + 1/2 ∠A`
`120° - 90° + 1/2 ∠A`
∠A = 2(30°)
∠A= 60°
Thus, ∠A = 60°
APPEARS IN
संबंधित प्रश्न
Can a triangle have two acute angles?Justify your answer in case.
In a triangle, an exterior angle at a vertex is 95° and its one of the interior opposite angle is 55°, then the measure of the other interior angle is
An exterior angle of a triangle is 108° and its interior opposite angles are in the ratio 4 : 5. The angles of the triangle are
State, if the triangle is possible with the following angles :
40°, 130°, and 20°
Find the value of the angle in the given figure:

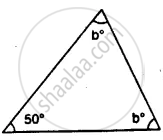
Find the unknown marked angles in the given figure:
One angle of a right-angled triangle is 70°. Find the other acute angle.
In ∆ABC, C = 56° C = 56° ∠B = ∠C and ∠A = 100° ; find ∠B.
The number of triangles in figure is ______.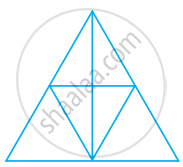
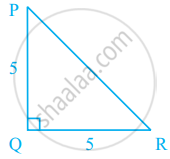
In figure, PQ ⊥ RQ, PQ = 5 cm and QR = 5 cm. Then ∆PQR is ______.