Advertisements
Advertisements
प्रश्न
In Fig. below, ABCD is a parallelogram in which ∠DAB = 75° and ∠DBC = 60°. Compute
∠CDB and ∠ADB.
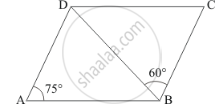
उत्तर

To find `∠`CDB and `∠`ADB
`∠`CBD = `∠`ABD = 60° [Alternative interior angle AD || BC and BD is the transversal]
In a parallelogram ABCD
`∠`A = `∠`C = 75° [ ∵ Opposite side angles of a parallelogram are equal]
In `∠`BDC
`∠`CBD + `∠`C + `∠`CDB =180° [Angle sum property]
⇒ 60° + 75° + `∠`CDB = 180°
⇒`∠`CDB = 180° - (60° + 75°)
⇒ `∠`CDB = 45°
Hence `∠`CDB = 45°, `∠`ADB = 60°
APPEARS IN
संबंधित प्रश्न
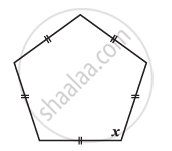
Find the angle measure x in the given Figure
Find x in the following figure:

In a quadrilateral ABCD, CO and DO are the bisectors of `∠`C and ∠D respectively. Prove that
`∠`COD = `1/2` (`∠`A+ `∠`B).
ABCD is a parallelogram in which ∠A = 70°. Compute ∠B, ∠C and ∠D .
Diagonals necessarily bisect opposite angles in a
The two diagonals are equal in a
The figure formed by joining the mid-points of the adjacent sides of a square is a
In ΔABC, ∠A = 30°, ∠B = 40° and ∠C = 110°. The angles of the triangle formed by joining the mid-points of the sides of this triangle are
The diagonals AC and BD of a rectangle ABCD intersect each other at P. If ∠ABD = 50°, then ∠DPC =
Three angles of a quadrilateral are equal. Fourth angle is of measure 120°. What is the measure of equal angles?
