Advertisements
Advertisements
प्रश्न
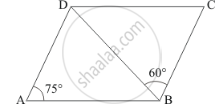
In Fig. below, ABCD is a parallelogram in which ∠DAB = 75° and ∠DBC = 60°. Compute
∠CDB and ∠ADB.
उत्तर

To find `∠`CDB and `∠`ADB
`∠`CBD = `∠`ABD = 60° [Alternative interior angle AD || BC and BD is the transversal]
In a parallelogram ABCD
`∠`A = `∠`C = 75° [ ∵ Opposite side angles of a parallelogram are equal]
In `∠`BDC
`∠`CBD + `∠`C + `∠`CDB =180° [Angle sum property]
⇒ 60° + 75° + `∠`CDB = 180°
⇒`∠`CDB = 180° - (60° + 75°)
⇒ `∠`CDB = 45°
Hence `∠`CDB = 45°, `∠`ADB = 60°
APPEARS IN
संबंधित प्रश्न
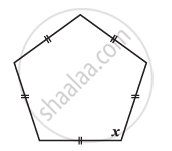
Find the angle measure x in the given Figure
If an angle of a parallelogram is two-third of its adjacent angle, find the angles of the parallelogram .
In the given figure, ABCD is a rectangle in which diagonal AC is produced to E. If ∠ECD = 146°, find ∠AOB.
If an angle of a parallelogram is two-third of its adjacent angle, the smallest angle of the parallelogram is
If the diagonals of a rhombus are 18 cm and 24 cm respectively, then its side is equal to
The diagonals AC and BD of a rectangle ABCD intersect each other at P. If ∠ABD = 50°, then ∠DPC =
Can all the angles of a quadrilateral be right angles? Give reason for your answer.
A quadrilateral has three acute angles. If each measures 80°, then the measure of the fourth angle is ______.
The angles P, Q, R and S of a quadrilateral are in the ratio 1:3:7:9. Then PQRS is a ______.
Sum of all the angles of a quadrilateral is 180°.
