Advertisements
Advertisements
प्रश्न
In figure, AB = BC and AD = BD = DC. The number of isosceles triangles in the figure is ______.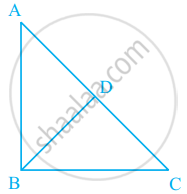
विकल्प
1
2
3
4
उत्तर
In figure, AB = BC and AD = BD = DC. The number of isosceles triangles in the figure is 3.
Explanation:
A triangle, in which two sides are equal, is known as an isosceles triangle.
Hence, there are 3 isosceles triangles in the given figure, i.e. ΔABC, ΔABD and ΔDBC .......[AB = BC, AD = DB and BD = DC]
APPEARS IN
संबंधित प्रश्न
Can a triangle have All angles less than 60° Justify your answer in case.
If each angle of a triangle is less than the sum of the other two, show that the triangle is acute angled.
Is the following statement true and false :
Sum of the three angles of a triangle is 180 .
In a triangle PQR, ∠P = 60° and ∠Q = ∠R, find ∠R.
Find the unknown marked angles in the given figure:
In ∆ABC, ∠A = ∠B = 62° ; find ∠C.
The length of the sides of the triangle is given. Say what types of triangles they are 4.3 cm, 4.3 cm, 4.3 cm.
The length of the sides of the triangle is given. Say what types of triangles they are 3.7 cm, 3.4 cm, 4 cm.
Can we have two acute angles whose sum is a right angle? Why or why not?
Which two triangles have ∠B in common?

