Advertisements
Advertisements
प्रश्न
In the following arrangement of capacitors, the energy stored in the 6 µF capacitor is E. Find the value of the following :
(i) Energy stored in 12 µF capacitor.
(ii) Energy stored in 3 µF capacitor.
(iii) Total energy drawn from the battery.
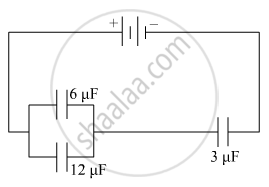
उत्तर
(i) Given that energy of the 6 µF capacitor is E.
Let V be the potential difference along the capacitor of capacitance 6 µF.
\[\text { Since } \frac{1}{2}C V^2 = E\]
\[ \therefore \frac{1}{2} \times 6 \times {10}^{- 6} \times V^2 = E\]
\[ \Rightarrow V^2 = \frac{E}{3} \times {10}^6 . . . (1)\]
Since potential is same for parallel connection, the potetial through 12 µF capacitor is also V. Hence, energy of 12 µF capacitor is
\[E_{12} = \frac{1}{2} \times 12 \times {10}^{- 6} \times V^2 = \frac{1}{2} \times 12 \times {10}^{- 6} \times \frac{E}{3} \times {10}^6 = 2E\]
(ii) Since charge remains constant in series, the charge on 6 µF and 12 µF capacitors combined will be equal to the charge on 3 µF capacitor. Using the formula, Q = CV, we can write
\[(6 + 12) \times {10}^{- 6} \times V = 3 \times {10}^{- 6} \times V'\]
\[\text { Using (1) and squaring both sides, we get }\]
\[V '^2 = 12E \times {10}^6 \]
\[ \therefore E_3 = \frac{1}{2} \times 3 \times {10}^{- 6} \times 12E \times {10}^6 = 18E\]
(iii) Total energy drawn from battery is
APPEARS IN
संबंधित प्रश्न
Explain what would happen if the capacitor given in previous question a 3 mm thick mica sheet (of dielectric constant = 6) were inserted between the plates,
- While the voltage supply remained connected.
- After the supply was disconnected.
A 12 pF capacitor is connected to a 50 V battery. How much electrostatic energy is stored in the capacitor?
How many time constants will elapse before the energy stored in the capacitor reaches half of its equilibrium value in a charging RC circuit?
A capacitor with stored energy 4⋅0 J is connected with an identical capacitor with no electric field in between. Find the total energy stored in the two capacitors.
A capacitor of capacitance 100 μF is connected across a battery of emf 6 V through a resistance of 20 kΩ for 4 s. The battery is then replaced by a thick wire. What will be the charge on the capacitor 4 s after the battery is disconnected?
Choose the correct option:
Energy stored in a capacitor and dissipated during charging a capacitor bear a ratio.
A capacitor is a device that stores ____________.
If the p. d. across a capacitor is increased from 10 V to 30 V, then the energy stored with the capacitor ____________.
A capacitor is charged by a battery and energy stored is 'U'. Now the battery is removed and the distance between plates is increased to four times. The energy stored becomes ______.
A 2µF capacitor is charge to 100 volt and then its plate are connected by a conducting wire. The heat produced is:-
