Advertisements
Advertisements
प्रश्न
In the given figure, ABCDE is a pentagon inscribed in a circle such that AC is a diameter and side BC//AE.If
∠ BAC=50°, find giving reasons:
(i) ∠ACB
(ii) ∠EDC
(iii) ∠BEC
Hence prove that BE is also a diameter

उत्तर

Let ∠ACB be x, ∠EDC be y and ∠BEC as z.
Now, ∠ABC = 90° {Angle in a semi-circle}
(i) ∴ In ΔABC, ∠ABC + ∠BAC + ∠ACB = 180°
⇒ 90° + 50° + ∠ACB = 180°
∠ACB = 40° = x
(ii) Now, ∠EAC = ∠ACB {Alternate interior angles ∴ AC is transversal to the parallel lines AE & BC}
∴ ∠EAC = 40°
Also, ∠EAC + ∠EDC = 180°
∴ 40° + ∠EDC = 180°
⇒ ∠EDC = 140° = y
(iii) ∠EBC + ∠EDC = 180° {Angles in cyclic quadrilateral}
∠EBC + 140° = 180°
∠EBC = 40°
∴ In ΔEBC
∠BEC + ∠ECB + ∠EBC = 180°
∴ ∠BEC + 90° + 40° = 180°
∴ ∠BEC = 50°
Also in ΔEAB
∠EAB = ∠EAC + ∠BAC
= 40° + 50°
∠EAB = 90°
We know, if an angle of a triangle in a circle is 90o then, the hypotenuse must be the diameter of the circle. Hence, BE is the diameter of the circle.
APPEARS IN
संबंधित प्रश्न
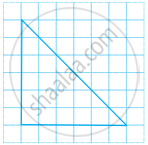
Complete the following figure, about the given lines of symmetry which are shown by dotted lines and name the figure thus obtained:
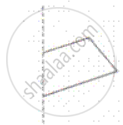
Given below are part of a geometrical figure. Complete the figure so that both axes are lines of symmetry.
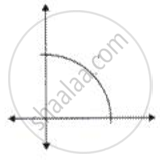
Construct an isosceles right-angled triangle, having hypotenuse= 8 cm. Draw its lines of symmetry.
Part of a geometrical figure is given in each of the diagrams below. Complete the figures so. that the line AB in each case is a line of symmetry of the completed figure.
Give also the geometrical name for the completed figure. Recognizable free hand Sketches would be awarded full marks.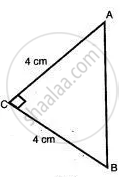
Draw the lines of symmetry of the following.
The number of capital letters of the English alphabets having only horizontal line of symmetry is ______.
A square and a rectangle have the same number of lines of symmetry.
Find the number of lines of symmetry for the following shape:
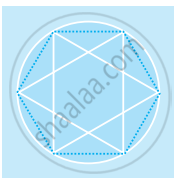
Find the number of lines of symmetry for the following shape:

Copy the triangle in the following figure on squared paper. Draw the line(s) of symmetry, if any and identify the type of triangle. (Some of you may like to trace the figure and try paper-folding first!)