Advertisements
Advertisements
प्रश्न
In the case given below, find the value of x so that POQ is straight line

उत्तर
POQ is a straight line.
In the given figure,
∵ POQ is a straight line
∴ ∠POL + ∠LOM + ∠MOQ = 180° (Straight line angles)
⇒ x + 20° + 2x - 30° + 3x - 50°= 180°
⇒ 6x + 20° - 80° = 180°
⇒ 6x - 60° = 180°
⇒ 6x = 180° + 60° = 240°
⇒ x = `240^circ/6`
⇒ x = 40°
∴ x = 40°
APPEARS IN
संबंधित प्रश्न
In your note-book copy the following angle using ruler and a pair compass only.
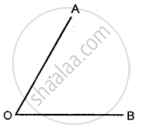
Construct the 135° angle, using ruler and a pair of compass only.
Draw line AB = 6 cm. Construct angle ABC = 60°. Then draw the bisector of angle ABC.
Draw a line segment AB = 7cm. Mark a point Pon AB such that AP=3 cm. Draw perpendicular on to AB at point P.
Draw a line segment OA = 5 cm. Use set-square to construct angle AOB = 60°, such that OB = 3 cm. Join A and B; then measure the length of AB.
Draw a line segment OP = 8cm. Use set-square to construct ∠POQ = 90°; such that OQ = 6 cm. Join P and Q; then measure the length of PQ.
In the following figures, PQ is parallel to RS. Find the angles a, b and c:
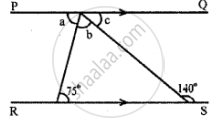
In the following figures, PQ is parallel to RS. Find the angles a, b and c:

Draw a line AB = 9 cm. Mark a point P in AB such that AP=5 cm. Through P draw (using set-square) perpendicular PQ = 3 cm. Measure BQ.
Without using set squares, construct angle ABC = 60° in which AB = BC = 5 cm. Join A and C and measure the length of AC.
