Advertisements
Advertisements
प्रश्न
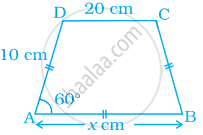
In the following figure, AB || DC and AD = BC. Find the value of x.
उत्तर
Given: An isosceles trapezium, AB || DC, AD = BC and ∠A = 60°.
So, ∠B = 60°.
Construction: Draw a line parallel to BC through D.
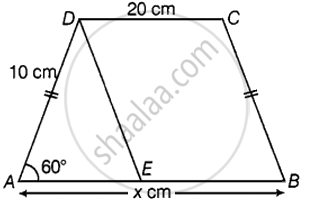
Now, DEBC is a parallelogram,
BE = CD = 20 cm and DE = BC = 10 cm.
Now, ∠DEB + ∠CBE = 180° ...[Adjacent angles are supplementary in parallelogram]
∠DEB = 180° – 60°
∠DEB = 120°
In triangle ADE,
∠ADE = 60° ...[Exterior angle]
Also, in triangle ADE is an equilateral triangle.
AE = 10 cm
AB = AE + EB
AB = 10 + 20
AB = 30 cm
Hence, x = 30 cm.
APPEARS IN
संबंधित प्रश्न
All parallelograms are trapeziums.
In `square` IJKL, side IJ || side KL, ∠I = 108°, ∠K = 53° then find the measure of ∠J and ∠L.
PQRS is a trapezium in which PQ || SR and ∠P = 130°, ∠Q = 110°. Then ∠R is equal to ______.
A quadrilateral in which a pair of opposite sides is parallel is ______.
In trapezium ABCD with AB || CD, if ∠A = 100°, then ∠D = ______.
All angles of a trapezium are equal.
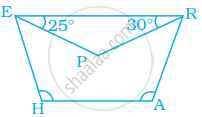
In trapezium HARE, EP and RP are bisectors of ∠E and ∠R respectively. Find ∠HAR and ∠EHA.
ABCD is a trapezium such that AB || CD, ∠A : ∠D = 2 : 1, ∠B : ∠C = 7 : 5. Find the angles of the trapezium.
Construct a trapezium ABCD in which AB || DC, ∠A = 105°, AD = 3 cm, AB = 4 cm and CD = 8 cm.
Construct a trapezium ABCD where AB || CD, AD = BC = 3.2 cm, AB = 6.4 cm and CD = 9.6 cm. Measure ∠B and ∠A.
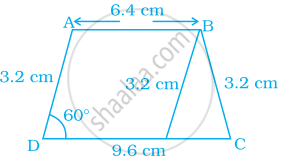
[Hint: Difference of two parallel sides gives an equilateral triangle.]
