Advertisements
Advertisements
प्रश्न
In the following figure, FD || BC || AE and AC || ED. Find the value of x.
Advertisements
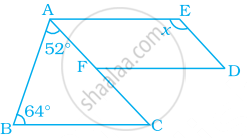
उत्तर
Given: FD || BC || AE and AC || ED.
Construction: Produce DF such that it intersect AB at G.
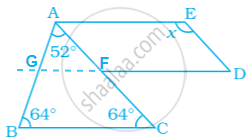
In triangle ABC,
∠A + ∠B + ∠C = 180° ...[Angle sum property of triangle]
52° + 64° + ∠C = 180°
∠C = 180° – (52° + 64°)
∠C = 180° – 116°
∠C = 64°
Now, as see that DG || BC and DG || AE,
∠ACB = ∠AFG ...[FG || BC and FC is a transversal. So, corresponding angles]
64° = ∠AFG
Also, GFD is a straight line.
So, ∠GFA + ∠AFD = 180° ...[Linear pair]
64° + ∠AFD = 180°
∠AFD = 180° – 64°
∠AFD = 116°
Also, FD || AE and AF || ED
Hence, AEDF is a parallelogram.
Now, ∠AFD = ∠AEF ...[Opposite angles in a parallelogram are equal]
∠AED = x = 116°
APPEARS IN
संबंधित प्रश्न
Referring the adjacent figure of a parallelogram, write the answer of questions given below.
(1) If l(WZ) = 4.5 cm then l(XY) = ?
(2) If l(YZ) = 8.2 cm then l(XW) = ?
(3) If l(OX) = 2.5 cm then l(OZ) = ?
(4) If l(WO) = 3.3 cm then l(WY) = ?
(5) If m∠WZY = 120° then m∠WXY = ? and m∠XWZ = ?
Given: Parallelogram ABCD in which diagonals AC and BD intersect at M.
Prove: M is the mid-point of LN.
In the following diagram, the bisectors of interior angles of the parallelogram PQRS enclose a quadrilateral ABCD.
Show that:
(i) ∠PSB + ∠SPB = 90°
(ii) ∠PBS = 90°
(iii) ∠ABC = 90°
(iv) ∠ADC = 90°
(v) ∠A = 90°
(vi) ABCD is a rectangle
Thus, the bisectors of the angles of a parallelogram enclose a rectangle.
In the given figure, AB || EC, AB = AC and AE bisects ∠DAC. Prove that:
- ∠EAC = ∠ACB
- ABCE is a parallelogram.
Iron rods a, b, c, d, e, and f are making a design in a bridge as shown in the figure. If a || b, c || d, e || f, find the marked angles between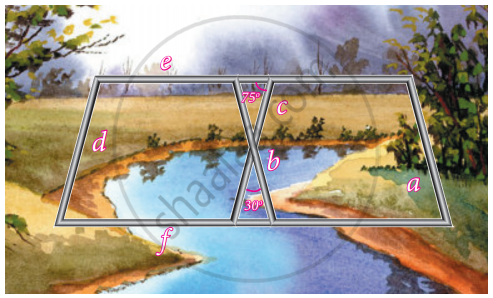 b and c
If opposite angles of a quadrilateral are equal, it must be a parallelogram.
In parallelogram PQRS, O is the mid point of SQ. Find ∠S, ∠R, PQ, QR and diagonal PR.
Two angles of a quadrilateral are each of measure 75° and the other two angles are equal. What is the measure of these two angles? Name the possible figures so formed.
ABCD is a parallelogram. The bisector of angle A intersects CD at X and bisector of angle C intersects AB at Y. Is AXCY a parallelogram? Give reason.
The angle between the two altitudes of a parallelogram through the vertex of an obtuse angle of the parallelogram is 45°. Find the angles of the parallelogram.
