Advertisements
Advertisements
प्रश्न
In the following figure, FD || BC || AE and AC || ED. Find the value of x.
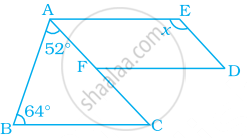
उत्तर
Given: FD || BC || AE and AC || ED.
Construction: Produce DF such that it intersect AB at G.
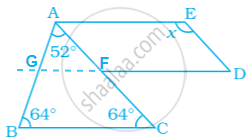
In triangle ABC,
∠A + ∠B + ∠C = 180° ...[Angle sum property of triangle]
52° + 64° + ∠C = 180°
∠C = 180° – (52° + 64°)
∠C = 180° – 116°
∠C = 64°
Now, as see that DG || BC and DG || AE,
∠ACB = ∠AFG ...[FG || BC and FC is a transversal. So, corresponding angles]
64° = ∠AFG
Also, GFD is a straight line.
So, ∠GFA + ∠AFD = 180° ...[Linear pair]
64° + ∠AFD = 180°
∠AFD = 180° – 64°
∠AFD = 116°
Also, FD || AE and AF || ED
Hence, AEDF is a parallelogram.
Now, ∠AFD = ∠AEF ...[Opposite angles in a parallelogram are equal]
∠AED = x = 116°
APPEARS IN
संबंधित प्रश्न
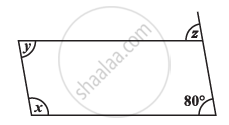
Consider the given parallelograms. Find the values of the unknowns x, y, z.
If the ratio of measures of two adjacent angles of a parallelogram is 1 : 2, find the measures of all angles of the parallelogram.
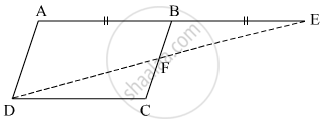
In the given figure, `square`ABCD is a parallelogram. Point E is on the ray AB such that BE = AB then prove that line ED bisects seg BC at point F.
Construct ☐ BARC such that l(BA) = l(BC) = 4.2 cm, l(AC) = 6.0 cm, l(AR) = l(CR) = 5.6 cm
The angle between the two altitudes of a parallelogram through the same vertex of an obtuse angle of the parallelogram is 30°. The measure of the obtuse angle is ______.
In the given figure, ABCD and BDCE are parallelograms with common base DC. If BC ⊥ BD, then ∠BEC = ______.
All rectangles are parallelograms.
If opposite angles of a quadrilateral are equal, it must be a parallelogram.
Construct a parallelogram when one of its side is 4 cm and its two diagonals are 5.6 cm and 7 cm. Measure the other side.
Draw a rough figure of a quadrilateral that is not a parallelogram but has exactly two opposite angles of equal measure.
