Question

In the given figure, seg AB is a diameter of a circle with centre O. The bisector of ∠ACB intersects the circle at point D. Prove that, seg AD ≅ seg BD.
Complete the following proof by filling in the blanks.

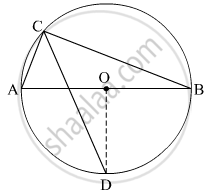
Proof: Draw seg OD.

∠ACB = ______      ...(Angle inscribed in semicircle)
∠DCB = ______      ...(CD is the bisector of ∠C)
m(arc DB) = ______   ...(Inscribed angle theorem)
∠DOB = ______      ...(Definition of measure of an arc)(I)

seg OA ≅ seg OB       ...( ____________ )(II)

∴ line OD is ______ of seg AB.     ...[From (I) and (II)]
∴ seg AD ≅ seg BD.

Answer 1

Given: Seg AB is a diameter of a circle with centre O. The bisector of ∠ACB intersects the circle at point D.

To Prove: seg AD ≅ seg BD.

Proof: Draw seg OD. 

∠ACB = 90°      ...(Angle inscribed in semicircle)
∠DCB = 45°      ...(CD is the bisector of ∠C)
m(arc DB) = 2∠DCB = 90°    ...(Inscribed angle theorem)
∠DOB = 90°      ...(Definition of measure of an arc)(I)

seg OA ≅ seg OB       ...( Radii of the circle )(II)

∴ line OD is perpendicular bisector of seg AB.     ...[From (I) and (II)]
∴ seg AD ≅ seg BD