Advertisements
Advertisements
प्रश्न
In the right angled Δ XYZ, ∠XYZ = 90° and a, b, c are the lengths of the sides as shown in the figure. Write the following ratios,
- sin X
- tan Z
- cos X
- tan X.

उत्तर
- sin X = `"Opposite side of ∠ X"/"Hypotenuse" = ["YZ"]/["XZ"] = a/c`
- tan Z = `"Opposite side of ∠ Z"/"Adjacent Side of ∠ Z" = ["XY"]/["YZ"] = b/a`
- cos X = `"Adjacent Side of ∠ X"/"Hypotenuse" = ["XY"]/["XZ"] = b/c`
- tan X = `" Opposite side of ∠X"/"Adjacent Side of ∠X" = "YZ"/"XY" = a/b`
APPEARS IN
संबंधित प्रश्न
In the given Figure, ∠R is the right angle of ΔPQR. Write the following ratios.
- sin P
- cos Q
- tan P
- tan Q

In right angled ΔLMN, ∠LMN = 90°, ∠L = 50° and ∠N = 40°, write the following ratios.
- sin 50°
- cos 50°
- tan 40°
- cos 40°

In the given figure, ∠PQR = 90°, ∠PQS = 90°, ∠PRQ = α and ∠QPS = θ Write the following trigonometric ratios.
- sin α, cos α, tan α
- sin θ, cos θ, tan θ

In the given figure, `angle` PQR = 90° , `angle` PQS = 90° , `angle` PRQ = θ and `angle` QPS = θ Write the following trigonometric ratios.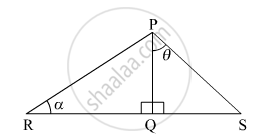
(i) sin θ, cos θ, tan θ
(ii) sin θ , cos θ , tan θ
In right angled ΔTSU, TS = 5, ∠S = 90°, SU = 12 then find sin T, cos T, tan T. Similarly find sin U, cos U, tan U.

In right angled ΔYXZ, ∠X = 90°, XZ = 8 cm, YZ = 17 cm, find sin Y, cos Y, tan Y, sin Z, cos Z, tan Z.

In right angled ΔLMN, if ∠N =θ, ∠M = 90, cos θ =`24/25`, find sin θ and tan θ Similarly, find (sin2 θ) and (cos2 θ).

