Advertisements
Advertisements
प्रश्न
In what ratio does the point Q(1, 6) divide the line segment joining the points P(2, 7) and R(−2, 3)
विकल्प
1 : 2
2 : 1
1 : 3
3 : 1
उत्तर
1 : 3
Explanation;
Hint:
A line divides internally in the ratio m : n the point P
= `(("m"x_2 + "n"x_1)/("m" + "n"), ("m"y_2 + "n"y_1)/("m" + "n"))`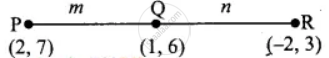
(1, 6) = `((-2"m" + 2"n")/("m" + "n"), (3"m" + 7"n")/("m" + "n"))`
= `(-2"m" + 2"n")/("m" + "n")` = 1
−2m + 2n = m + nx
−3m = n – 2n
3m = n
`"m"/"n" = 1/3`
∴ m : n = 1 : 3
and
`(3"m" + 7"n")/("m" + "n")` = 6
3m + 7n = 6m + 6n
6m – 3n = 7n – 6n
3m = n
`"m"/"n" = 1/3`
APPEARS IN
संबंधित प्रश्न
The points (2, –1), (–1, 4) and (–2, 2) are mid-points of the sides of a triangle. Find its vertices.
Find the coordinates of the midpoint of the line segment joining P(0, 6) and Q(12, 20).
Find the midpoint of the line segment joining the following pair of point :
( a+3, 5b), (3a-1, 3b +4).
Find the length of the median through the vertex A of triangle ABC whose vertices are A (7, -3), B(S, 3) and C(3, -1).
A( 4, 2), B(-2, -6) and C(l, 1) are the vertices of triangle ABC. Find its centroid and the length of the median through C.
A(3, 1), B(y, 4) and C(1, x) are vertices of a triangle ABC. P, Q and R are mid - points of sides BC, CA and AB respectively. Show that the centroid of ΔPQR is the same as the centroid ΔABC.
The midpoint of the line segment joining (2a, 4) and (-2, 2b) is (1, 2a+1). Find the value of a and b.
show that the points A(- 1, 2), B(2, 5) and C(- 5, – 2) are collinear.
The points A(−3, 6), B(0, 7) and C(1, 9) are the mid-points of the sides DE, EF and FD of a triangle DEF. Show that the quadrilateral ABCD is a parallelogram.
The mid-point of the line joining (−a, 2b) and (−3a, −4b) is
If A(5, 4), B(–3, –2) and C(1, –8) are the vertices of a ∆ABC. Segment AD is median. Find the length of seg AD:
