Advertisements
Advertisements
प्रश्न
The points A(−3, 6), B(0, 7) and C(1, 9) are the mid-points of the sides DE, EF and FD of a triangle DEF. Show that the quadrilateral ABCD is a parallelogram.
उत्तर
Let D be (x1 y1), E(x2, y2) and F(x3, y3)

Mid−point of a line = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
Mid−point of DE = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
(−3, 6) = `((x_1 + x_2)/2, (y_1 + y_2)/2)`
x1 + x2 = −6 → (1)
y1 + y2 = 12 → (2)
Mid−point of EF = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
(0, 7) = `((x_2 + x_3)/2, (y_2 + y_3)/2)`
∴ x2 + x3 = 0 → (3)
y2 + y3 = 14 → (4)
Mid−point of FD = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
(1, 9) = `((x_1 + x_3)/2, (y_1 + y_3)/2)`
x1 + x3 = 2 → (5)
y1 + y3 = 18 → (6)
Add (1) + (3) + (5)
2x1 + 2x2 + 2x3 = −6 + 0 + 2
2(x1 + x2 + x3) = −4
x1 + x2 + x3 = −2
From (1) x1 + x2 = −6
x3 = −2 + 6 = 4
From (3) x2 + x3 = 0
x1 = −2 + 0
x1 = −2
From (5) x1 + x3 = 2
x2 + 2 = −2
x2 = −2 − 2 = −4
∴ x1 = −2, x2 = −4, x3 = 4
Add (2) + (4) + 6
2y1 + 2y2 + 2y3 = 12 + 14 + 18
2(y1 + y2 + y3) = 44
y1 + y2 + y3 = `44/2` = 22
From (2) y1 + y2 = 12
12 + y3 = 22
⇒ y3 = 22 – 12
y3 = 10
From (4) y2 + y3 = 14
y1 + 14 = 22
⇒ y1 = 22 – 14
y1 = 8
From (6)
y1 + y3 = 18
y2 + 18 = 22
⇒ = 22 – 18
y2 = 4
∴ y1 = 8, y2 = 4, y3 = 10
The vertices D is (−2, 8)
Mid−point of AC = `((-3 + 1)/2, (6 + 9)/2)`
= `(-1, 15/2)`
Mid−point of BD = `((0 - 2)/2, (7 + 8)/2)`
= `((-2)/2, 15/2)`
= `(-1, 15/2)`
Mid-point of diagonal AC = Mid-point of diagonal BD
The quadrilateral ABCD is a parallelogram.
APPEARS IN
संबंधित प्रश्न
Calculate the co-ordinates of the centroid of the triangle ABC, if A = (7, –2), B = (0, 1) and C =(–1, 4).
Write the co-ordinates of the point of intersection of graphs of
equations x = 2 and y = -3.
Find the midpoint of the line segment joining the following pair of point :
( -3, 5) and (9, -9)
If the midpoints of the sides ofa triangle are (-2, 3), (4, -3), (4, 5), find its vertices.
Two vertices of a triangle are ( -1, 4) and (5, 2). If the centroid is (0, 3), find the third vertex.
A , B and C are collinear points such that AB = `1/2` AC . If the coordinates of A, B and C are (-4 , -4) , (-2 , b) anf (a , 2),Find the values of a and b.
AB is a diameter of a circle with centre 0. If the ooordinates of A and 0 are ( 1, 4) and (3, 6 ). Find the ooordinates of B and the length of the diameter.
Find coordinates of midpoint of the segment joining points (0, 2) and (12, 14)
If A(5, 4), B(–3, –2) and C(1, –8) are the vertices of a ∆ABC. Segment AD is median. Find the length of seg AD:
Point P is the centre of the circle and AB is a diameter. Find the coordinates of points B if coordinates of point A and P are (2, – 3) and (– 2, 0) respectively.
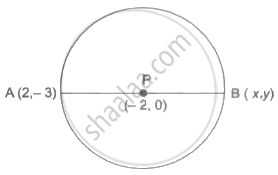
Given: A`square` and P`square`. Let B (x, y)
The centre of the circle is the midpoint of the diameter.
∴ Mid point formula,
`square = (square + x)/square`
⇒ `square = square` + x
⇒ x = `square - square`
⇒ x = – 6
and `square = (square + y)/2`
⇒ `square` + y = 0
⇒ y = 3
Hence coordinates of B is (– 6, 3).
