Advertisements
Advertisements
प्रश्न
Prove that the points A (a,0), B( 0,b) and C (1,1) are collinear, if `( 1/a+1/b) =1`.
उत्तर
Consider the points A (a,0), B( 0,b) and C (1,1) .
` Here (x_1=a,y_1=0).(x_2 = 0,y_2=b) and (x_3=1,y_3=1).`
It is given that the points are collinear. So,
`x_1 (y_2-y_3)+x_2(y_3-y_1) +x_3(y_1-y_2) =0`
`⇒ a(b-1)+0(1-0)+1(0-b)=0`
`⇒ ab-a-b=0`
Dividing the equation by ab:
`⇒ 1-1/b-1/a=0`
`⇒ 1-(1/a+1/b)=0`
`⇒(1/a+1/b)=1`
Therefore, the given points are collinear if `(1/a+1/b)=1`
APPEARS IN
संबंधित प्रश्न
In each of the following find the value of 'k', for which the points are collinear.
(8, 1), (k, -4), (2, -5)
Find the area of a triangle with vertices at the point given in the following:
(1, 0), (6, 0), (4, 3)
The four vertices of a quadrilateral are (1, 2), (−5, 6), (7, −4) and (k, −2) taken in order. If the area of the quadrilateral is zero, find the value of k.
The area of a triangle is 5. Two of its vertices are (2, 1) and (3, −2). The third vertex lies on y = x + 3. Find the third vertex.
Show that the points A(-5,6), B(3,0) and C(9,8) are the vertices of an isosceles right-angled triangle. Calculate its area.
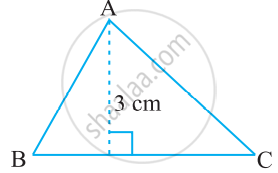
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
If the sides of a triangle are 3 cm, 4 cm and 5 cm, then the area is
The table given below contains some measures of the right angled triangle. Find the unknown values.
| Base | Height | Area |
| ? | 12 m | 24 sq.m |
If the co-ordinates of the vertices of an equilateral triangle with sides of length ‘a’ are (x1, y1), (x2, y2), (x3, y3), then `|(x_1, y_1, 1),(x_2, y_2, 1),(x_3, y_3, 1)|^2 = (3"a"^4)/4`
If the points (2, -3), (k, -1), and (0, 4) are collinear, then find the value of 4k.
