Advertisements
Advertisements
प्रश्न
Ruler and compasses may be used in this question. All construction lines and arcs must be clearly shown and be of sufficient length and clarity to permit assessment.
- Construct a ΔABC, in which BC = 6 cm, AB = 9 cm and angle ABC = 60°.
- Construct the locus of all points inside triangle ABC, which are equidistant from B and C.
- Construct the locus of the vertices of the triangles with BC as base and which are equal in area to triangle ABC.
- Mark the point Q, in your construction, which would make ΔQBC equal in area to ΔABC, and isosceles.
- Measure and record the length of CQ.
Advertisements
उत्तर
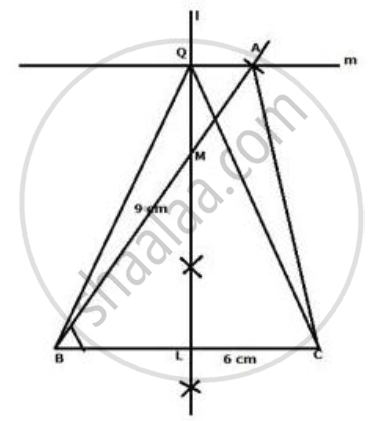
Steps of construction:
- Draw a line segment BC = 6 cm.
- At B, draw a ray BX making an angle 60 degree and cut off BA = 9 cm.
- Join AC. ABC is the required triangle.
- Draw perpendicular bisector of BC which intersects BA in M, then any point on LM is equidistant from B and C.
- Through A, draw a line m || BC.
- The perpendicular bisector of BC and the parallel line m intersect each other at Q.
- Then triangle QBC is equal in area to triangle ABC. m is the locus of all points through which any triangle with base BC will be equal in area of triangle ABC.
On measuring CQ = 8.4 cm.
APPEARS IN
संबंधित प्रश्न
On a graph paper, draw the lines x = 3 and y = –5. Now, on the same graph paper, draw the locus of the point which is equidistant from the given lines.
Construct an isosceles triangle ABC such that AB = 6 cm, BC = AC = 4 cm. Bisect ∠C internally and mark a point P on this bisector such that CP = 5 cm. Find the points Q and R which are 5 cm from P and also 5 cm from the line AB.
Plot the points A(2, 9), B(–1, 3) and C(6, 3) on graph paper. On the same graph paper draw the locus of point A so that the area of ΔABC remains the same as A moves.
Draw two intersecting lines to include an angle of 30°. Use ruler and compasses to locate points which are equidistant from these Iines and also 2 cm away from their point of intersection. How many such points exist?
Without using set squares or protractor, construct a quadrilateral ABCD in which ∠ BAD = 45°, AD = AB = 6 cm, BC= 3.6 cm and CD=5 cm. Locate the point P on BD which is equidistant from BC and CD.
Draw and describe the lorus in the following cases:
The locus of points at a distance of 4 cm from a fixed line.
Draw and describe the locus in the following case:
The locus of a point in rhombus ABCD which is equidistant from AB and AD.
Describe completely the locus of a point in the following case:
Centre of a circle of varying radius and touching the two arms of ∠ ABC.
Construct a triangle ABC, such that AB= 6 cm, BC= 7.3 cm and CA= 5.2 cm. Locate a point which is equidistant from A, B and C.
Ruler and compasses only may be used in this question. All construction lines and arcs must be clearly shown, and be of sufficient length and clarity to permit assessment.
(i) Construct a ΔABC, in which BC = 6 cm, AB = 9 cm and ∠ABC = 60°.
(ii) Construct the locus of the vertices of the triangles with BC as base, which are equal in area to ΔABC.
(iii) Mark the point Q, in your construction, which would make ΔQBC equal in area to ΔABC, and isosceles.
(iv) Measure and record the length of CQ.
