Advertisements
Advertisements
प्रश्न
Show that the following points are collinear:
A(8,1), B(3, -4) and C(2, -5)
उत्तर
`"Let" A(x_1=8,y_1=1), B (x_2=3, y_2=-4) and C (x_3 = 2, y_3=-5) ` be the given points.
Now `x_1(y_2-y_3)+x_2(y_3-y_1)+x_3(y_1-y_2)`
=8 (-4+5) +3 (-5-1) +2(1+4)
= 8 - 18 + 10
=0
Hence, the given points are collinear.
APPEARS IN
संबंधित प्रश्न
median of a triangle divides it into two triangles of equal areas. Verify this result for ΔABC whose vertices are A (4, - 6), B (3, - 2) and C (5, 2).
Find values of k if area of triangle is 4 square units and vertices are (−2, 0), (0, 4), (0, k)
For what value of a point (a, 1), (1, -1) and (11, 4) are collinear?
In a ΔABC, AB = 15 cm, BC = 13 cm and AC = 14 cm. Find the area of ΔABC and hence its altitude on AC ?
Find the area of Δ ABC whose vertices are:
A (1,2) B (-2,3) and C (-3,-4)
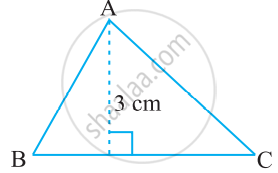
Find BC, if the area of the triangle ABC is 36 cm2 and the height AD is 3 cm.
The area of the triangle whose vertices are A(1, 2), B(-2, 3) and C(-3, -4) is ______.
The points (1,1), (-2, 7) and (3, -3) are ______.
Find the cost of laying grass in a triangular field of sides 50 m, 65 m and 65 m at the rate of Rs 7 per m2.
In the given figure, ratio of the area of triangle ABC to the area of triangle ACD is the same as the ratio of base BC of triangle ABC to the base CD of ΔACD.