Question

Solve the following system of equations by cross-multiplications method.

`a(x + y) + b (x – y) = a^2 – ab + b^2`

`a(x + y) – b (x – y) = a^2 + ab + b^2`

Answer 1

The given system of equations can be rewritten as

`ax + bx + ay – by – ( a^2 – ab + b^2 ) = 0`

`⇒ (a + b) x + (a – b) y – (a^2 – ab + b^2 ) = 0 ….(1)`

`a(x + y) – b (x – y) = a^2 + ab + b^2`

`⇒ (a – b) x + (a + b) y – (a^2 + ab + b^2 ) = 0 …(2)`

Now, by cross-multiplication method, we have

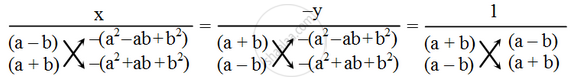
`\Rightarrow \frac{x}{(a-b)\times\{-(a^{2}+ab+b^{2})\}-(a+b)\times\{-(a^{2}-ab+b^{2})}\}=\frac{-y}{(a+b)\times\{-(a^{2}+ab+b^{2})\}-(a-b)\times\{-(a^{2}-ab+b^{2})\}}=\frac{1}{(a+b)\times (a+b)-(a-b)(a-b)}`

`\Rightarrow\frac{x}{-(a-b)(a^{2}+ab+b^{2})+(a+b)(a^{2}-ab+b^{2})}=\frac{-y}{-(a+b)(a^{2}+ab+b^{2})+(a-b)(a^{2}-ab+b^{2})}=\frac{1}{(a+b)^{2}-(a-b)^{2}`

`\Rightarrow\frac{x}{-(a^{3}-b^{3})+(a^{3}+b^{2})}=\frac{-y}{-a^{3}-2a^{2}b-2ab^{2}-b^{3}+a^{3}-2a^{2}b+2ab^{2}-b^{3}}=\frac{1}{a^{2}+2ab+b^{2}-a^{2}+2ab-b^{2}`

`\Rightarrow\frac{x}{2b^{3}}=\frac{-y}{-4a^{2}b-2b^{3}}=\frac{1}{4ab}`

`\Rightarrow\frac{x}{2b^{3}}=\frac{-y}{-2b(2a^{2}+b^{2})}=\frac{1}{4ab}`

`\Rightarrow \frac{x}{2b^{3}}=\frac{1}{4ab}\Rightarrowx=\frac{b^{2}}{2a}`

`and\text{ }\frac{-y}{-2b(2a^{2}+b^{2})}=\frac{1}{4ab}\Rightarrowy=\frac{2a^{2}+b^{2}}{2a}`

Hence, the solution is `x=\frac{b^{2}}{2a},y=\frac{2a^{2}+b^{2}}{2a}`