Advertisements
Advertisements
प्रश्न
Solve the following inequalities and represent the solution on a number line:
3x + 4 ≤ x + 8
उत्तर
We have, 3x + 4 ≤ x + 8
⇒ 3x - x ≤ 8 - 4 ...[Bring like terms on one side]
⇒ 2x ≤ 4
⇒ x ≤ 2
The graph of the solution set is x ≤ 2.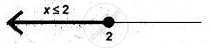
APPEARS IN
संबंधित प्रश्न
Represent the following inequalities on real number line:
–5 < x ≤ –1
Given A = {x : –1 < x ≤ 5, x ∈ R} and B = {x : – 4 ≤ x < 3, x ∈ R}
Represent on different number lines:
A – B
Given that x ∈ I. solve the inequation and graph the solution on the number line:
`3 >= (x - 4)/2 + x/3 >= 2`
Solve the following inequation and represent the solution set on the number line:
`4x - 19 < (3x)/5 - 2 <= (-2)/5 + x, x ∈ R`
Solve the following inequation, write the solution set and represent it on the number line.
`-3 (x - 7) ≥ 15 - 7x > (x + 1)/3, x ∈ R`
Give that x ∈ I. Solve the inequation and graph the solution on the number line:
`3≥(x - 4)/(2)+x/(3)≥2`
Graph the solution set for each inequality:
x ≥ - 3
Solve : `(4x - 10)/(3) ≤ (5x - 7)/(2)` x ∈ R and represent the solution set on the number line.
Solve the following inequation, write the solution set and represent it on the real number line.
`5x - 21 < (5x)/7 - 6 ≤ -3 3/7 + x, x ∈ R`
The following number line represents:

