Advertisements
Advertisements
प्रश्न
Solve the following inequation and represent the solution set on the number line:
`-3 < -1/2 - (2x)/3 ≤ 5/6, x ∈ R`
उत्तर
`-3 < -1/2 - (2x)/3 ≤ 5/6`
Multiply by 6, we get
`=> -18 < -3 - 4x ≤ 5`
`=> -15 < - 4x ≤ 8`
Dividing by – 4, we get
`=> (-15)/(-4) > x ≥ 8/(-4)`
`=> -2 ≤ x < 15/4`
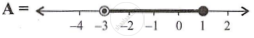
`=> x ∈ [-2,15/4]`
The solution set can be represented on a number line as:
![]()
APPEARS IN
संबंधित प्रश्न
Represent the following inequalities on real number line:
– 4 < x < 4
Represent the following inequalities on real number line:
–5 < x ≤ –1
Represent the solution of the following inequalities on the real number line:
4x – 1 > x + 11
Given: A = {x : –8 < 5x + 2 ≤ 17, x ∈ I}, B = {x : –2 ≤ 7 + 3x < 17, x ∈ R}
Where R = {real numbers} and I = {integers}. Represent A and B on two different number lines. Write down the elements of A ∩ B.
Find the set of values of x, satisfying:
`7x + 3 >= 3x - 5` and `x/4 - 5 <= 5/4 -x`, where x ∈ N
Solve the following inequation and represent the solution set on the number line:
`4x - 19 < (3x)/5 - 2 <= (-2)/5 + x, x ∈ R`
Solve the following inequalities and represent the solution on a number line:
4 - 2x ≥ 6 - 3x
Solve the following inequalities and represent the solution on a number line:
3(x - 2) > 1
Solve the inequation 2x – 5 ≤ 5x + 4 < 11, where x ∈ I. Also represent the solution set on the number line.
The real number lines for two inequations A and B are as given below, A ∩ B is: