Advertisements
Advertisements
प्रश्न
Solve the following quadratic equation by
factorisation.
5m2 = 22m + 15
उत्तर
5m2 = 22m + 15
\[\Rightarrow 5 m^2 - 22m - 15 = 0\]
\[ \Rightarrow 5 m^2 - 25m + 3m - 15 = 0\]
\[ \Rightarrow 5m\left( m - 5 \right) + 3\left( m - 5 \right) = 0\]
\[ \Rightarrow \left( 5m + 3 \right)\left( m - 5 \right) = 0\]
\[ \Rightarrow \left( 5m + 3 \right) = 0 or \left( m - 5 \right) = 0\]
\[ \Rightarrow m = \frac{- 3}{5} \text{ or } m = 5\]
So, \[\frac{- 3}{5} \text{ and } 5\] are the roots of the given quadratic equation.
APPEARS IN
संबंधित प्रश्न
Solve for x : `(x+1)/(x-1)+(x-1)/(x+2)=4-(2x+3)/(x-2);x!=1,-2,2`
Find the roots of the following quadratic equation by factorisation:
`sqrt2 x^2 +7x+ 5sqrt2 = 0`
A cottage industry produces a certain number of pottery articles in a day. It was observed on a particular day that the cost of production of each article (in rupees) was 3 more than twice the number of articles produced on that day. If the total cost of production on that day was Rs 90, find the number of articles produced and the cost of each article.
Solve the following quadratic equations by factorization:
`x^2-(sqrt2+1)x+sqrt2=0`
Let us find two natural numbers which differ by 3 and whose squares have the sum 117.
Solve the following quadratic equations by factorization: \[\frac{x + 1}{x - 1} + \frac{x - 2}{x + 2} = 4 - \frac{2x + 3}{x - 2}; x \neq 1, - 2, 2\]
Find the value of k for which the following equations have real and equal roots:
\[\left( k + 1 \right) x^2 - 2\left( k - 1 \right)x + 1 = 0\]
Find the value of p for which the quadratic equation
\[\left( p + 1 \right) x^2 - 6(p + 1)x + 3(p + 9) = 0, p \neq - 1\] has equal roots. Hence, find the roots of the equation.
Disclaimer: There is a misprinting in the given question. In the question 'q' is printed instead of 9.
If a and b are roots of the equation x2 + ax + b = 0, then a + b =
Three years ago, a man was 5 times the age of his son. Four years hence, he will be thrice his son's age. Find the present ages of the man and his son.
The area of the isosceles triangle is 60 cm2, and the length of each one of its equal side is 13cm. Find its base.
Solve equation using factorisation method:
(x + 3)2 – 4(x + 3) – 5 = 0
Solve the following quadratic equation by factorisation:
x2 - 3x - 10 = 0
Solve the following equation by factorization
(x – 4)2 + 52 = 132
Find three consecutive odd integers, the sum of whose squares is 83.
Find the roots of the following quadratic equation by the factorisation method:
`3sqrt(2)x^2 - 5x - sqrt(2) = 0`
In the centre of a rectangular lawn of dimensions 50 m × 40 m, a rectangular pond has to be constructed so that the area of the grass surrounding the pond would be 1184 m2 [see figure]. Find the length and breadth of the pond.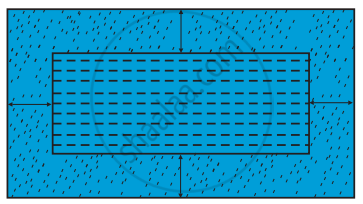
Solve the quadratic equation: x2 – 2ax + (a2 – b2) = 0 for x.
If the discriminant of the quadratic equation 3x2 - 2x + c = 0 is 16, then the value of c is ______.
(x – 3) (x + 5) = 0 gives x equal to ______.
